
CHAPTER 9
Chemical Quantities
1.
The coefficients of the balanced chemical equation for a reaction give the relative numbers of
molecules of reactants and products that are involved in the reaction.
2.
The coefficients of this balanced chemical equation indicate the relative numbers of molecules (or
moles) of each reactant that combine, as well as the number of molecules (or moles) of the
product formed.
3.
Although we define mass as the “amount of matter in a substance,” the units in which we
measure mass are a human invention. Atoms and molecules react on an individual particle-by-
particle basis, and we have to count individual particles when doing chemical calculations.
4.
(e); Subscripts cannot be changed to balance the equation or else the identities of the reactants
and/or product are changed. The balanced equation is: N2(g) + 3H2(g) 2NH3(g). Nitrogen and
hydrogen will react regardless of how much is initially present (just one might be used up before
the other).
5.
a.
PCl3(l) + 3H2O(l) H3PO3(aq) + 3HCl(g)
One molecule of liquid phosphorus trichloride reacts with three molecules of liquid
water, producing one molecule of aqueous phosphorous acid and molecules of gaseous
hydrogen chloride. One mole of phosphorus trichloride reacts with three moles of water
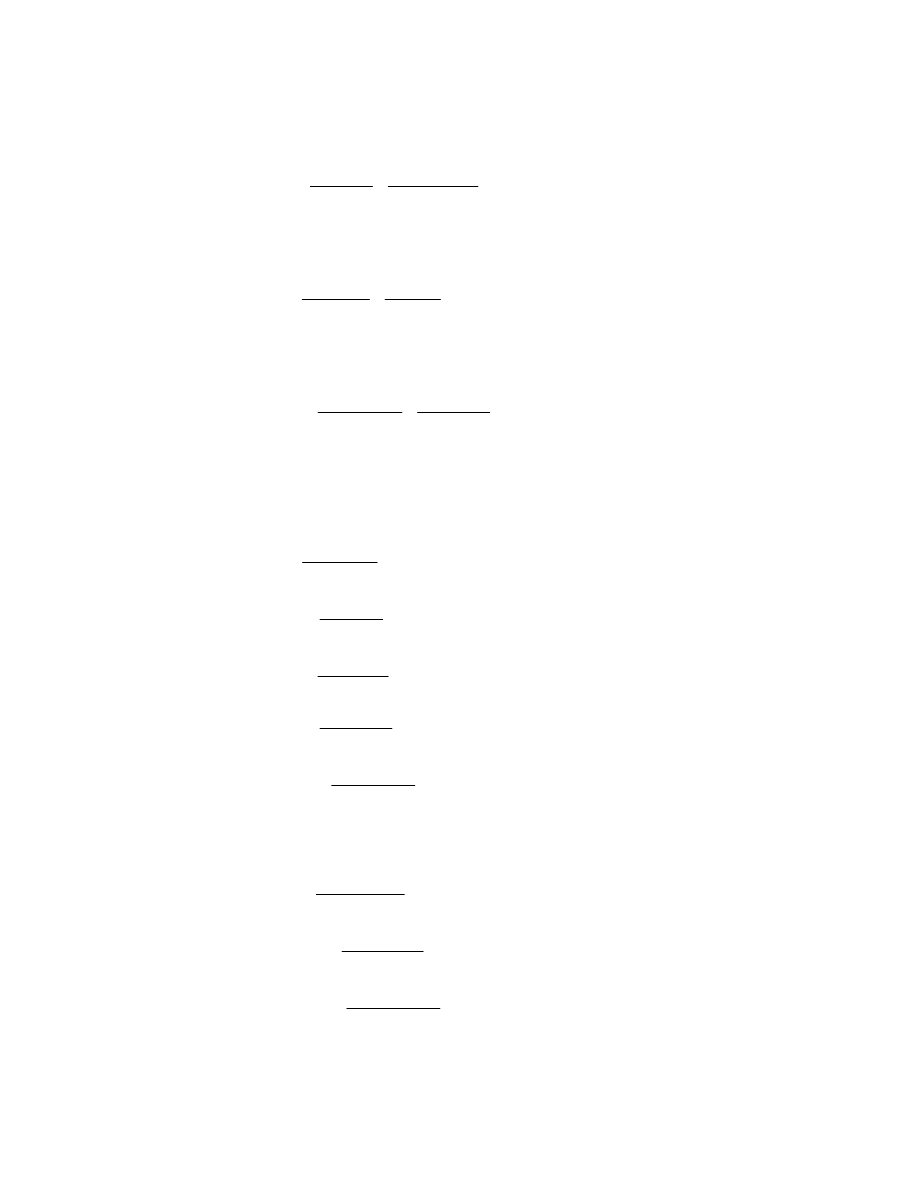
to produce one mole of phosphorous acid and three moles of hydrogen chloride.
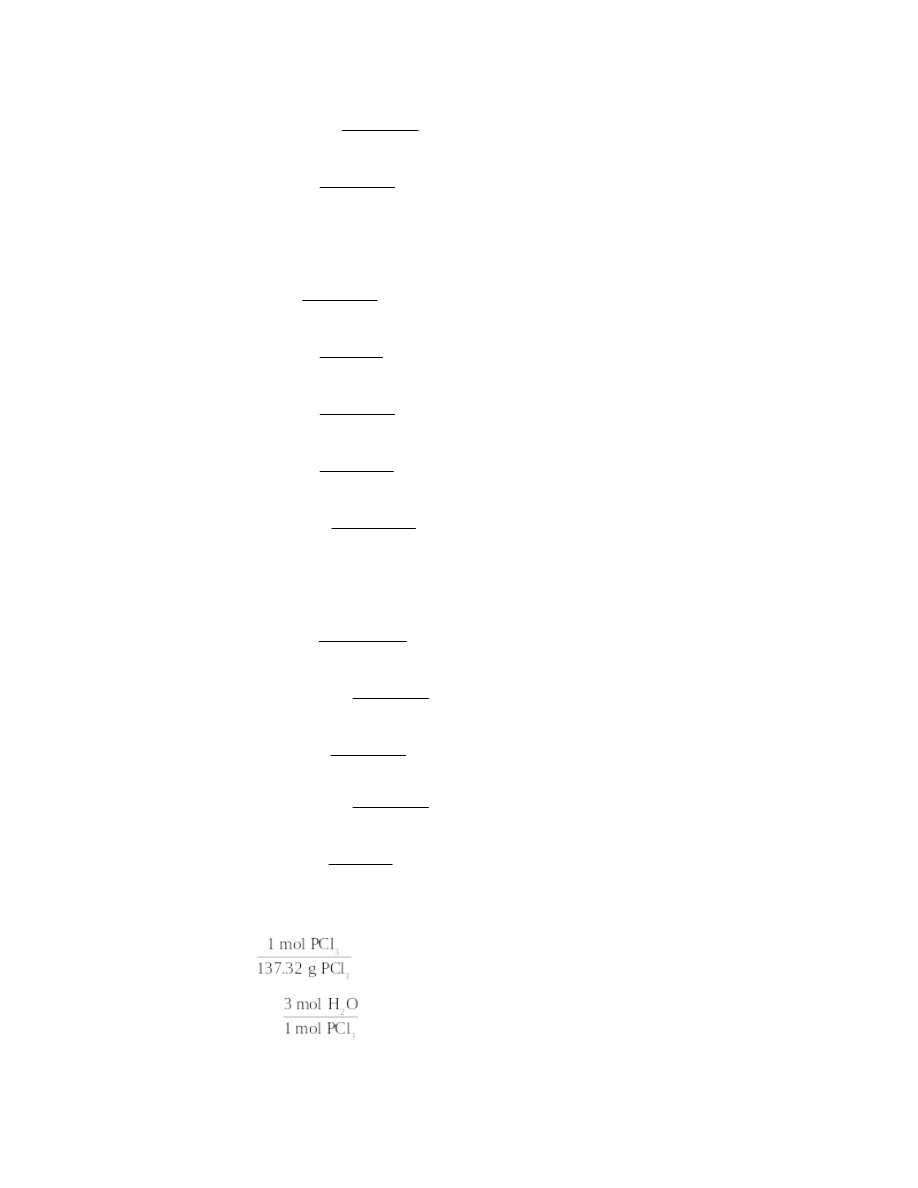
b.
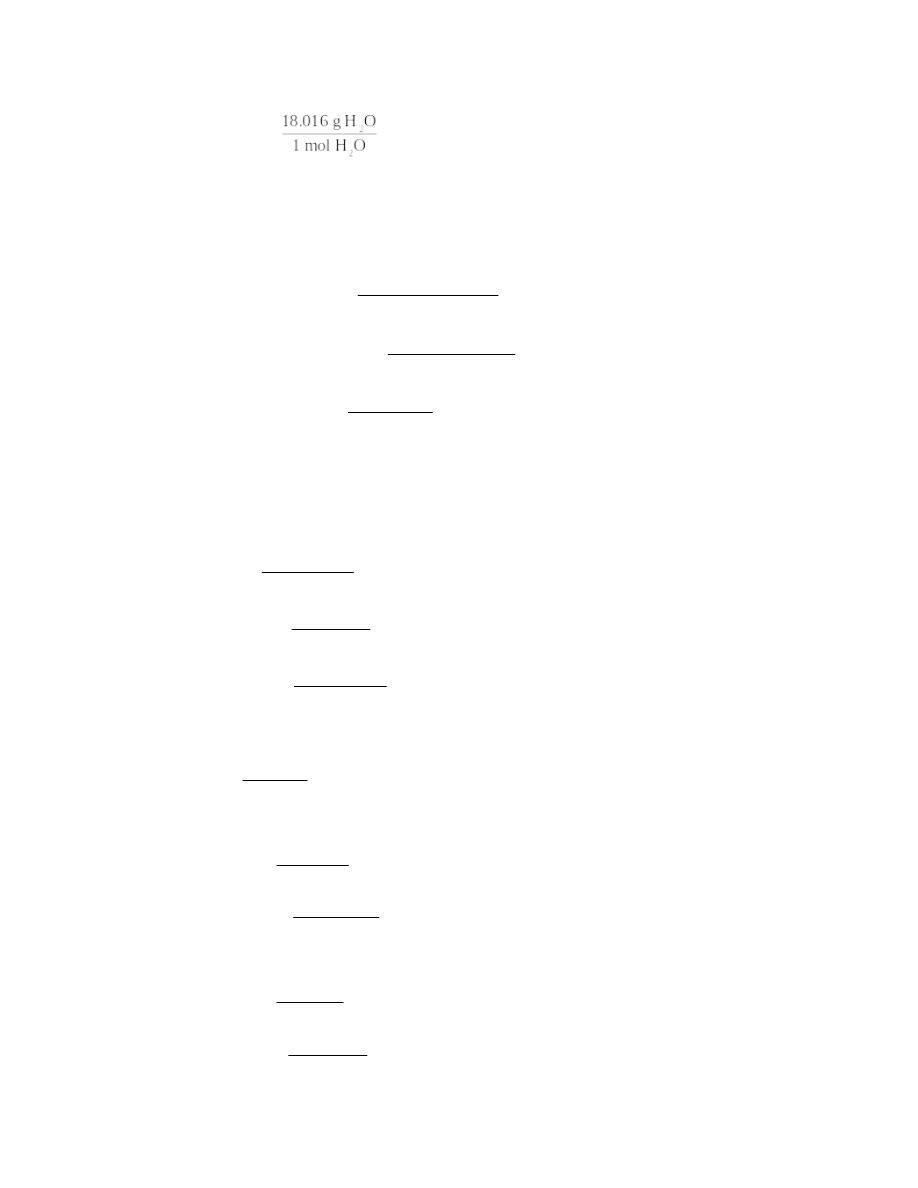
2XeF2(g) + 2H2O(l) 2Xe(g) + 4HF(g) + O2(g)
Two molecules of gaseous xenon difluoride react with two molecules of liquid water,
producing two gaseous xenon atoms, four molecules of gaseous hydrogen fluoride, and
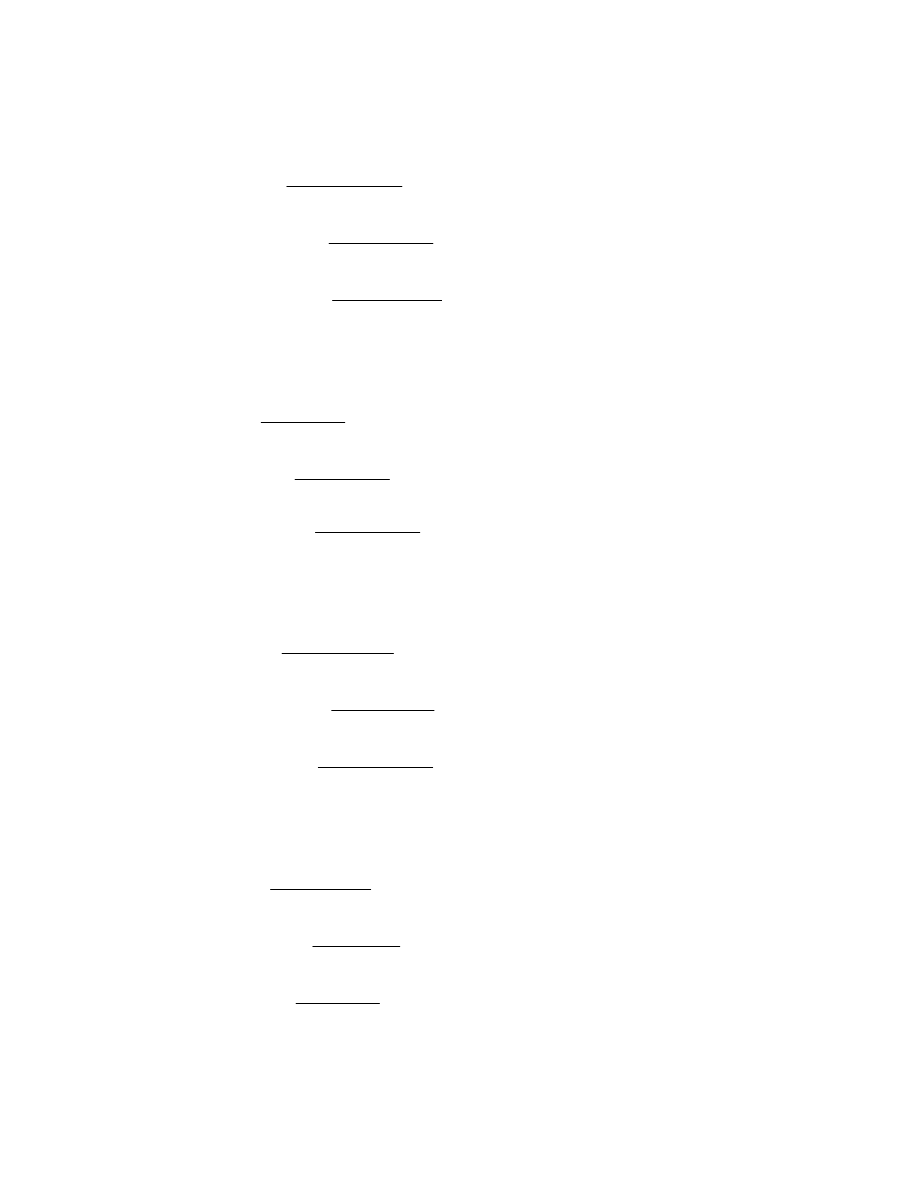
one molecule of oxygen gas. Two moles of xenon difluoride reacts with two moles of
water, to produce two moles of xenon, four moles of hydrogen fluoride, and one mole of
oxygen.
c.
S(s) + 6HNO3(aq) H2SO4(aq) + 2H2O(l) + 6NO2(g)
One sulfur atom reacts with six molecules of aqueous nitric acid, producing one molecule
of aqueous sulfuric acid, two molecules of water, and six molecules of nitrogen dioxide
gas. One mole of sulfur reacts with six moles of nitric acid, to produce one mole of
sulfuric acid, two moles of water, and six moles of nitrogen dioxide.
d.
2NaHSO3(s) Na2SO3(s) + SO2(g) + H2O(l)
Two formula units of solid sodium hydrogen sulfite react to produce one formula unit of
solid sodium sulfite, one molecule of gaseous sulfur dioxide, and one molecule of liquid
water. Two moles of sodium hydrogen sulfite react to produce one mole of sodium
sulfite, one mole of sulfur dioxide, and one mole of water.
163
Chapter 9: Chemical Quantities
6.
a.
3MnO2(s) + 4Al(s) 3Mn(s) + 2Al2O3(s)
Three formula units (or three moles) of manganese(IV) oxide react with four atoms (or
four moles) of aluminum, producing three atoms (or three moles) of manganese and two
formula units (or two moles) of aluminum oxide.
b.
B2O3(s) + 3CaF2(s) 2BF3(g) + 3CaO(s)
One molecule (or one mole) of diboron trioxide reacts with three formula units (or three
moles) of calcium fluoride, producing two molecules (or two moles) of boron trifluoride
and three formula units (three moles) of calcium oxide.
c.
3NO2(g) + H2O(l) 2HNO3(aq) + NO(g)
Three molecules (or three moles) of nitrogen dioxide react with one molecule (or one
mole) of water, producing two molecules (or two moles) of nitric acid and one molecule
(or one mole) of nitrogen monoxide.
d.
C6H6(g) + 3H2(g) C6H12(g)
One molecule (or one mole) of benzene (C6H6) reacts with three molecules (or three
moles) of hydrogen gas, producing one molecule (or one mole) of cyclohexane (C6H12).
7.
False. The coefficients of the balanced chemical equation represent the ratios on a mole basis by
which carbon combines with oxygen.
8.
Balanced chemical equations tell us in what molar ratios substances combine to form products,
not in what mass proportions they combine. How could a total of 3 g of reactants produce 2 g of
product?
9.
4Al(s) + 3O2(g) 2Al2O3(s)
For converting from a given number of moles of aluminum metal to the number of moles of
oxygen needed for reaction, the correct mole ratio is
2
3 mol O
4 mol Al
For converting from a given number of moles of aluminum metal to the number of moles of
product produced, the mole ratio is
2
3
2 mol Al O
4 mol Al
10.
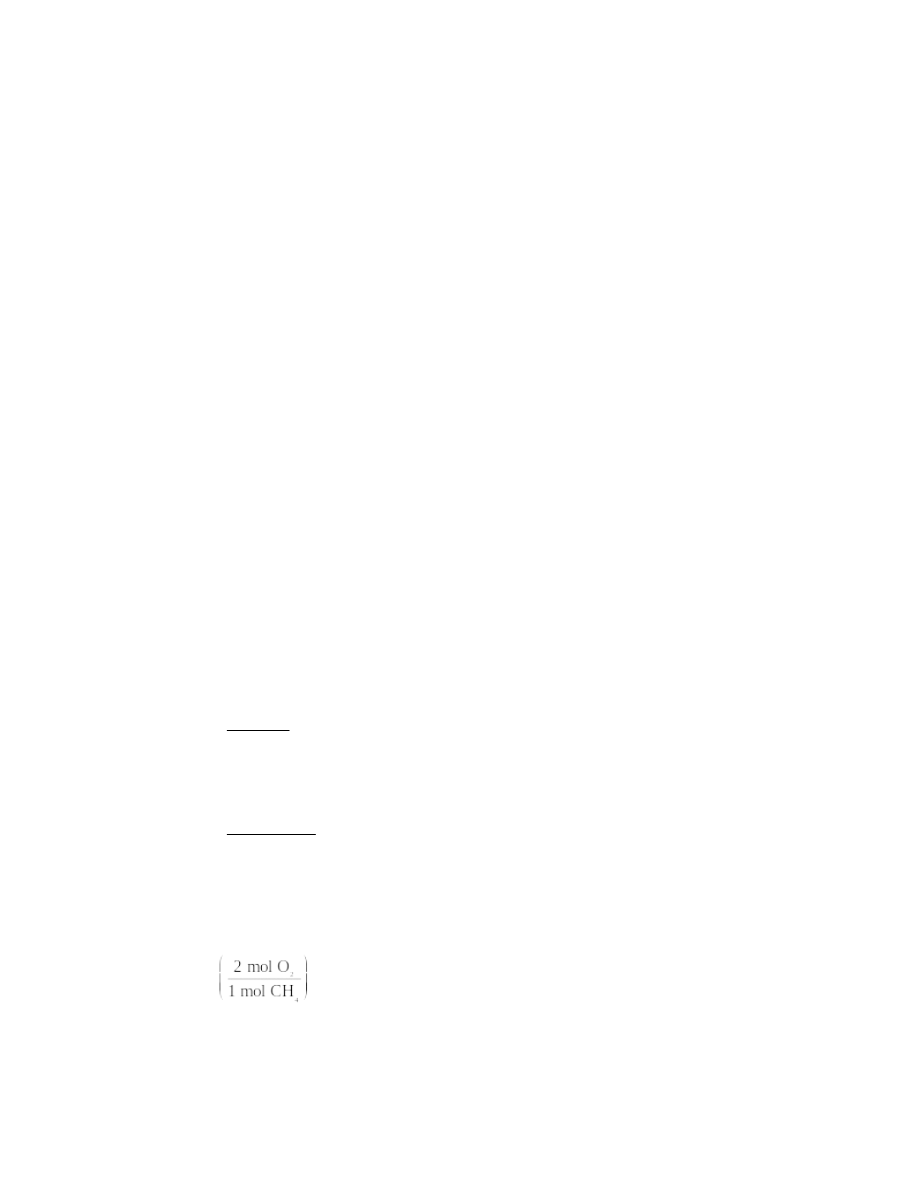
CH4(g) + 2O2(g) CO2(g) + 2H2O(g)
For converting from a given number of moles of methane to the number of moles of oxygen
required, the mole ratio is
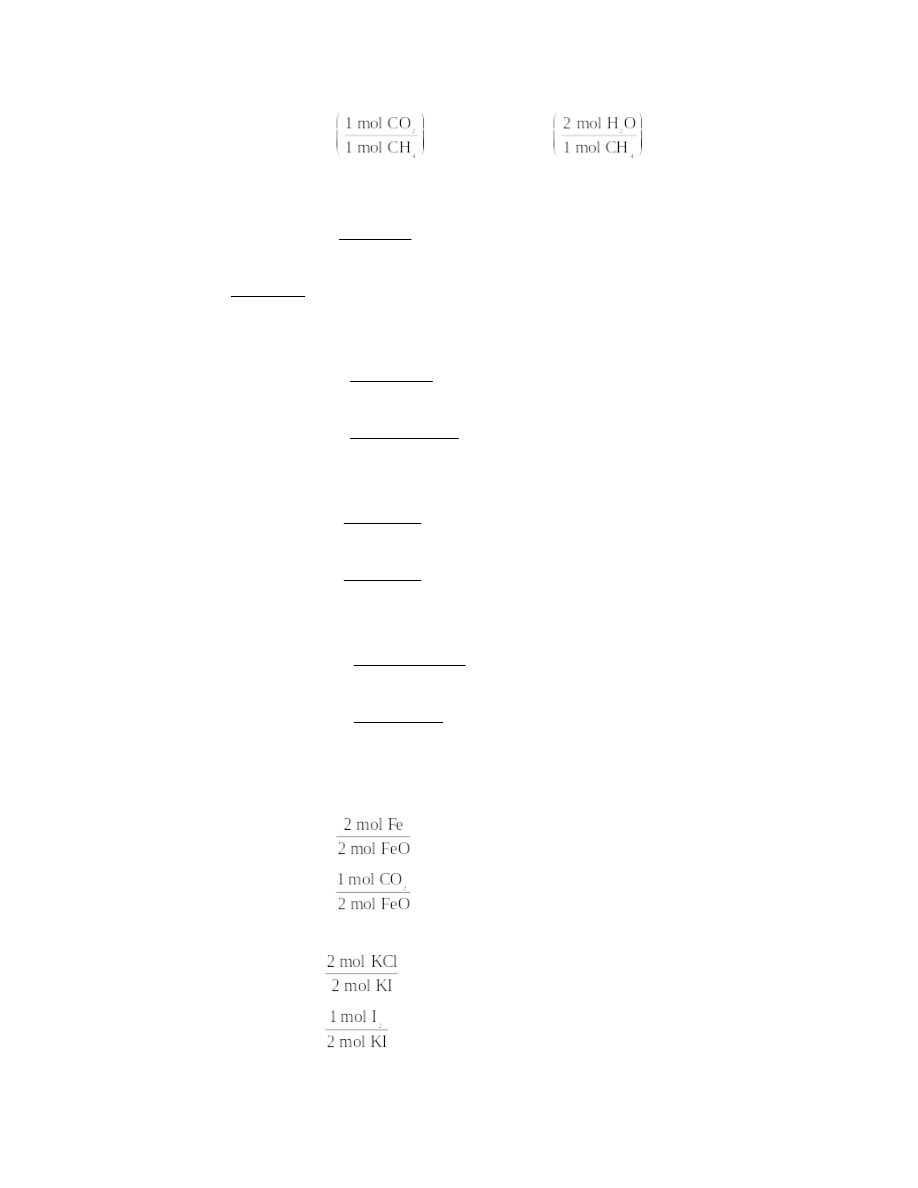
For a given number of moles of methane reacting completely, the mole ratios used to
calculate the number of moles of each product are
164
Chapter 9: Chemical Quantities
For CO2:
For H2O:
11.
a.
CO2(g) + 4H2(g) CH4(g) + 2H2O(l)
0.500 mol CO2
4
2
1 mol CH
1 mol CO
= 0.500 mol CH4
0.500 mol CO2
2
2
2 mol H O
1 mol CO
= 1.00 mol H2O
b.
BaCl2(aq) + 2AgNO3(aq) 2AgCl(s) + Ba(NO3)2(aq)
0.500 mol BaCl2
2
2 mol AgCl
1 mol BaCl
= 1.00 mol AgCl
0.500 mol BaCl2
3 2
2
1 mol Ba(NO )
1 mol BaCl
= 0.500 mol Ba(NO3)2
c.
C3H8(g) + 5O2(g) 4H2O(l) + 3CO2(g)
0.500 mol C3H8
2
3
8
4 mol H O
1 mol C H
= 2.00 mol H2O
0.500 mol C3H8
2
3
8
3 mol CO
1 mol C H
= 1.50 mol CO2
d.
3H2SO4(aq) + 2Fe(s) Fe2(SO4)3(aq) + 3H2(g)
0.500 mol H2SO4
2
4 3
2
4
1 mol Fe (SO )
3 mol H SO
= 0.167 mol Fe2(SO4)3
0.500 mol H2SO4
2
2
4
3 mol H
3 mol H SO
= 0.500 mol H2
12.
Before doing the calculations, the equations must be balanced.
a.
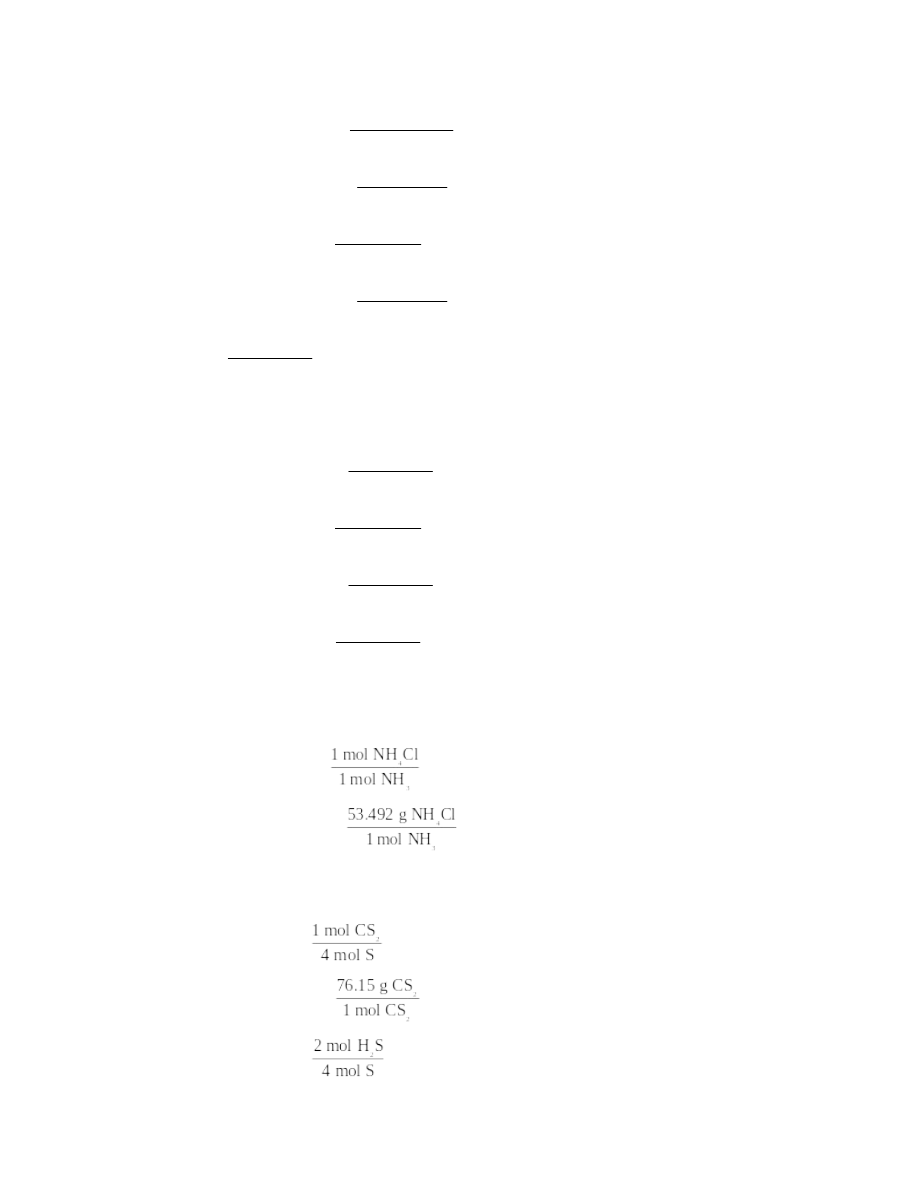
2FeO(s) + C(s) 2Fe(l) + CO2(g)
0.125 mol FeO ×
= 0.125 mol Fe
0.125 mol FeO ×
= 0.0625 mol CO2
b.
Cl2(g) + 2KI(aq) 2KCl(aq) + I2(s)
0.125 mol KI ×
= 0.125 mol KCl
0.125 mol KI ×
= 0.0625 mol I2
c.
2Na2B4O7(s) + 2H2SO4(aq) + 10H2O(l) 8H3BO3(s) + 2Na2SO4(aq)
165

Chapter 9: Chemical Quantities
0.125 mol Na2B4O7 ×
= 0.500 mol H3BO3
0.125 mol Na2B4O7 ×
= 0.125 mol Na2SO4
d.
CaC2(s) + 2H2O(l) Ca(OH)2(s) + C2H2(g)
0.125 mol CaC2 ×
= 0.125 mol Ca(OH)2
0.125 mol CaC2 ×
= 0.125 mol C2H2
13.
a.
AgNO3(aq) + LiOH(aq) AgOH(s) + LiNO3(aq)
molar masses: AgOH, 124.91 g; LiNO3, 68.95 g
0.125 mol AgNO3
3
1 mol AgOH
1 mol AgNO
= 0.125 mol AgOH
0.125 mol AgOH ×
124.91 g AgOH
1 mol AgOH
= 15.6 g AgOH
0.125 mol AgNO3
3
3
1 mol LiNO
1 mol AgNO
= 0.125 mol LiNO3
0.125 mol LiNO3 ×
3
3
68.95 g LiNO
1 mol LiNO
= 8.62 g LiNO3
b.
Al2(SO4)3(aq) + 3CaCl2(aq) 2AlCl3(aq) + 3CaSO4(s)
molar masses: AlCl3, 133.33 g; CaSO4, 136.15 g
0.125 mol Al2(SO4)3
3
2
4 3
2 mol AlCl
1 mol Al (SO )
= 0.250 mol AlCl3
0.250 mol AlCl3 ×
3
3
133.33 g AlCl
1 mol AlCl
= 33.3 g AlCl3
0.125 mol Al2(SO4)3
4
2
4 3
3 mol CaSO
1 mol Al (SO )
= 0.375 mol CaSO4
0.375 mol CaSO4 ×
4
136.15 g CaSO4
1 mol CaSO
= 51.1 g CaSO4
c.
CaCO3(s) + 2HCl(aq) CaCl2(aq) + CO2(g) + H2O(l)
molar masses: CaCl2, 110.98 g; CO2, 44.01 g; H2O, 18.02 g
0.125 mol CaCO3
2
3
1 mol CaCl
1 mol CaCO
= 0.125 mol CaCl2
166
Chapter 9: Chemical Quantities
0.125 mol CaCl2
2
2
110.98 g CaCl
1 mol CaCl
= 13.9 g CaCl2
0.125 mol CaCO3
2
3
1 mol CO
1 mol CaCO
= 0.125 mol CO2
0.125 mol CO2 ×
2
2
44.01 g CO
1 mol CO
= 5.50 g CO2
0.125 mol CaCO3
2
3
1 mol H O
1 mol CaCO
= 0.125 mol H2O
0.125 mol H2O ×
2
2
18.02 g H O
1 mol H O
= 2.25 g H2O
d.
2C4H10(g) + 13O2(g) 8CO2(g) + 10H2O(g)
molar masses: CO2, 44.01 g; H2O, 18.02 g
0.125 mol C4H10
2
4
10
8 mol CO
2 mol C H
= 0.500 mol CO2
0.500 mol CO2 ×
2
2
44.01 g CO
1 mol CO
= 22.0 g CO2
0.125 mol C4H10
2
4
10
10 mol H O
2 mol C H
= 0.625 mol H2O
0.625 mol H2O ×
2
2
18.02 g H O
1 mol H O
= 11.3 g H2O
14.
a.
NH3(g) + HCl(g) NH4Cl(s)
molar mass: NH4Cl, 53.492 g
0.50 mol NH3
= 0.50 mol NH4Cl
0.50 mol NH4Cl
= 27 g NH4Cl
b.
CH4(g) + 4S(s) CS2(l) + 2H2S(g)
molar masses: CS2, 76.15 g; H2S, 34.086 g
0.50 mol S
= 0.125 mol CS2
0.125 mol CS2
= 9.5 g CS2
0.50 mol S
= 0.25 mol H2S
167

Chapter 9: Chemical Quantities
0. 25 mol H2S
= 8.5 g H2S
c.
PCl3(l) + 3H2O(l) H3PO3(aq) + 3HCl(aq)
molar masses: H3PO3, 81.994 g; HCl, 36.458 g
0.50 mol PCl3
= 0.50 mol H3PO3
0.50 mol H3PO3
= 41 g H3PO3
0.50 mol PCl3
= 1.50 mol HCl
1.50 mol HCl
= 55 g HCl
d.
NaOH(s) + CO2(g) NaHCO3(s)
molar masses: NaHCO3, 84.008 g
0.50 mol NaOH
= 0.50 mol NaHCO3
0.50 mol NaHCO3
= 42 g NaHCO3
15.
a.
Cl2(g) + 2KI(aq) 2KCl(aq) + I2(s)
0.275 mol Cl2
2
2 mol KI
1 mol Cl
= 0.550 mol KI
b.
6Co(s) + P4(s) 2Co3P2(s)
0.275 mol Co
4
1 mol P
6 mol Co
= 0.0458 mol P4
c.
Zn(s) + 2HNO3(aq) Zn(NO3)2(aq) + H2(g)
0.275 mol Zn
3
2 mol HNO
1 mol Zn
= 0.550 mol HNO3
168
Chapter 9: Chemical Quantities
d.
C5H12(l) + 8O2(g) 5CO2(g) + 6H2O(g)
0.275 mol C5H12
2
5
12
8 mol O
1 mol C H
= 2.20 mol O2
16.
Before doing the calculations, the equations must be balanced.
a.
4KO2(s) + 2H2O(l) 3O2(g) + 4KOH(s)
0.625 mol KOH
2
3 mol O
4 mol KOH
= 0.469 mol O2
b.
SeO2(g) + 2H2Se(g) 3Se(s) + 2H2O(g)
0.625 mol H2O
2
3 mol Se
2 mol H O
= 0.938 mol Se
c.
2CH3CH2OH(l) + O2(g) 2CH3CHO(aq) + 2H2O(l)
0.625 mol H2O
3
2
2 mol CH CHO
2 mol H O
= 0.625 mol CH3CHO
d.
Fe2O3(s) + 2Al(s) 2Fe(l) + Al2O3(s)
0.625 mol Al2O3
2
3
2 mol Fe
1 mol Al O
= 1.25 mol Fe
17.
the molar mass of the substance
18.
Stoichiometry is the process of using a chemical equation to calculate the relative masses (or
moles) of reactants and products involved in a reaction.
19.
a.
molar mass Si = 28.09 g
4.15 g Si ×
1 mol Si
28.09 g Si
= 0.148 mol Si
b.
molar mass AuCl3 = 303.4 g
2.72 mg AuCl3 ×
3
3
1 mol AuCl
1 g
1000 mg
303.4 g AuCl
= 8.97 × 10–6 mol AuCl3
c.
molar mass S = 32.07 g
1.05 kg S ×
1000 g
1 mol S
1 kg
32.07 g S
= 32.7 mol S
d.
molar mass FeCl3 = 162.20 g
0.000901 g FeCl3 ×
3
3
1 mol FeCl
162.2 g FeCl
= 5.55 × 10–6 mol FeCl3
169

Chapter 9: Chemical Quantities
e.
molar mass MgO = 40.31 g
5.62 × 103 g MgO ×
1 mol MgO
40.31 g MgO
= 139 mol MgO
20.
a.
molar mass Ag = 107.9 g
2.01 × 10–2 g Ag ×
= 1.86 × 10–4 mol Ag
b.
molar mass (NH4)2S = 68.154 g
45.2 mg (NH4)2S ×
×
= 6.63 × 10–4 mol (NH4)2S
c.
molar mass U = 238.00 g
61.7
U ×
×
= 2.59 × 10–7 mol U
d.
molar mass SO2 = 64.07 g
5.23 kg SO2 ×
×
= 81.6 mol SO2
e.
molar mass Fe(NO3)3 = 241.88 g
272 g Fe(NO3)3 ×
= 1.12 mol Fe(NO3)3
21.
a.
molar mass Ge = 72.59 g
2.17 mol Ge
72.59 g Ge
1 mol Ge
= 158 g Ge
b.
molar mass PbCl2 = 278.1 g;
4.24 millimol = 0.00424 mol
0.00424 mol PbCl2
2
2
278.1 g PbCl
1 mol PbCl
= 1.18 g PbCl2
c.
molar mass NH3 = 17.03 g
0.0971 mol NH3
3
3
17.03 g NH
1 mol NH
= 1.65 g NH3
d.
molar mass C6H14 = 86.17 g
4.26 × 103 mol C6H14
6
14
6
14
86.17 g C H
1 mol C H
= 3.67 × 105 g C6H14
e.
molar mass ICl = 162.35 g
1.71 mol ICl
162.35 g ICl
1 mol ICl
= 278 g ICl
170

Chapter 9: Chemical Quantities
22.
a.
molar mass of K3N = 131.31 g
0.341 mol K3N
= 44.8 g K3N
b.
molar mass of Ne = 20.18 g; 2.62 millimol = 0.00262 mol
0.00262 mol Ne
= 0.0529 g Ne
c.
molar mass of MnO = 70.94 g
0.00449 mol MnO
= 0.319 g MnO
d.
molar mass of SiO2 = 60.09 g
7.18 × 105 mol SiO2
= 4.31 × 107 g SiO2
e.
molar mass of FePO4 = 150.82 g
0.000121 mol FePO4
= 0.0182 g FePO4
23.
Before any calculations are done, the equations must be balanced.
a.
2Co(s) + 3F2(g) 2CoF3(s)
0.413 mol Co ×
2
3 mol F
2 mol Co
= 0.620 mol F2
b.
2Al(s) + 3H2SO4(aq) Al2(SO4)3(aq) + 3H2(g)
0.413 mol Al ×
2
4
3 mol H SO
2 mol Al
= 0.620 mol H2SO4
c.
2K(s) + 2H2O(l) 2KOH(aq) + H2(g)
0.413 mol K ×
2
2 mol H O
2 mol K
= 0.413 mol H2O
d.
4Cu(s) + O2(g) 2Cu2O(s)
0.413 mol Cu ×
2
1 mol O
4 mol Cu
= 0.103 mol O2
24.
Before any calculations are done, the equations must be balanced.
a.
2Al(s) + 3Br2(l) 2AlBr3(s)
molar mass Al = 26.98 g
0.557 g Al ×
2
3 mol Br
1 mol Al
26.98 g Al
2 mol Al
= 0.0310 mol Br2
171
Chapter 9: Chemical Quantities
b.
Hg(s) + 2HClO4(aq) Hg(ClO4)2(aq) + H2(g)
molar mass Hg = 200.6 g
0.557 g Hg ×
4
2 mol HClO
1 mol Hg
200.6 g
1 mol Hg
= 0.00555 mol HClO4
c.
3K(s) + P(s) K3P(s)
molar mass K = 39.10 g
0.557 g K ×
1 mol K
1 mol P
39.10 g K 3 mol K
= 0.00475 mol P
d.
CH4(g) + 4Cl2(g) CCl4(l) + 4HCl(g)
molar mass CH4 = 16.04 g
0.557 g CH4 ×
4
2
4
4
1 mol CH
4 mol Cl
16.04 g CH
1 mol CH
= 0.139 mol Cl2
25.
Before any calculations are done, the equations must be balanced.
a.
TiBr4(g) + 2H2(g) Ti(s) + 4HBr(g)
molar mass H2 = 2.016 g; molar mass Ti = 47.90 g; molar mass of HBr = 80.91 g
12.5 g H2
2
2
1 mol H
2.016 g H
= 6.20 mol H2
6.20 mol H2
2
1 mol Ti
2 mol H
= 3.10 mol Ti
3.10 mol Ti
47.90 g Ti
1 mol Ti
= 148 g Ti
6.20 mol H2
2
4 mol HBr
2 mol H
= 12.4 mol HBr
12.4 mol HBr
80.91 g HBr
1 mol HBr
= 1.00 103 g HBr
b.
3SiH4(g) + 4NH3(g) Si3N4(s) + 12H2(g)
molar mass SiH4 = 32.12 g; molar mass Si3N4 = 140.3 g; molar mass H2 = 2.016 g
12.5 g SiH4
4
4
1 mol SiH
32.12 g SiH
= 0.389 mol SiH4
0.389 mol SiH4
3
4
4
1 mol Si N
3 mol SiH
= 0.130 mol Si3N4
0.130 mol Si3N4
3
4
3
4
140.3 g Si N
1 mol Si N
= 18.2 g Si3N4
172
Chapter 9: Chemical Quantities
0.389 mol SiH4
2
4
12 mol H
3 mol SiH
= 1.56 mol H2
1.56 mol H2
2
2
2.016 g H
1 mol H
= 3.14 g H2
c.
2NO(g) + 2H2(g) N2(g) + 2H2O(l)
molar mass H2 = 2.016 g; molar mass N2 = 28.02 g; molar mass H2O = 18.02 g
12.5 g H2
2
2
1 mol H
2.016 g H
= 6.20 mol H2
6.20 mol H2
2
2
1 mol N
2 mol H
= 3.10 mol N2
3.10 mol N2
2
2
28.02 g N
1 mol N
= 86.9 g N2
6.20 mol H2
2
2
2 mol H O
2 mol H
= 6.20 mol H2O
6.20 mol H2O
2
2
18.02 g H O
1 mol H O
= 112 g H2O
d.
Cu2S(s) 2Cu(s) + S(g)
molar mass Cu2S = 159.2 g; molar mass Cu = 63.55 g; molar mass S = 32.07 g
12.5 g Cu2S
2
2
1 mol Cu S
159.2 g Cu S
= 0.0785 mol Cu2S
0.0785 mol Cu2S
2
2 mol Cu
1 mol Cu S
= 0.157 mol Cu
0.157 mol Cu
63.55 g Cu
1 mol Cu
= 9.98 g Cu
0.0785 mol Cu2S
2
1 mol S
1 mol Cu S
= 0.0785 mol S
0.0785 mol S
32.07 g S
1 mol S
= 2.52 g S
26.
molar masses: PCl3, 137.32 g; H2O, 18.016 g
20.0 g PCl3 ×
= 0.146 mol PCl3
0.146 mol PCl3 ×
= 0.437 mol H2O
173
Chapter 9: Chemical Quantities
0.437 mol H2O ×
= 7.87 g H2O
27.
The equation must first be balanced.
(NH4)2CO3(s) 2NH3(g) + CO2(g) + H2O(g
molar masses: (NH4)2CO3, 96.09 g; NH3, 17.03 g
1.25 g (NH4)2CO3
4
3
2
4
3
2
1 mol NH
CO
96.09 g NH
CO
= 0.0130 mol (NH4)2CO3
0.0130 mol (NH4)2CO3 ×
3
4
3
2
2 mol NH
1 mol NH
CO
= 0.0260 mol NH3
0.0260 mol NH3
3
3
17.03 g NH
1 mol NH
= 0.443 g NH3
28.
The balanced equation for the reaction is:
CaC2(s) + 2H2O(l) C2H2(g) + Ca(OH)2(s)
molar masses: CaC2, 64.10 g; C2H2, 26.04 g
3.75 g CaC2 ×
2
2
1 mol CaC
64.10 g CaC
= 0.0585 mol CaC2
0.0585 mol CaC2×
2
2
2
1 mol C H
1 mol CaC
= 0.0585 mol C2H2
0.0585 mol C2H2 ×
2
2
2
2
26.04 g C H
1 mol C H
= 1.52 g C2H2
29.
molar masses: C, 12.01 g; CO, 28.01 g; CO2, 44.01 g
5.00 g C
1 mol C
12.01 g C
= 0.4163 mol C
carbon dioxide: C(s) + O2(g) CO2(g)
0.4163 mol C
2
1 mol CO
1 mol C
= 0.4163 mol CO2
0.4163 mol CO2
2
2
44.01 g CO
1 mol CO
= 18.3 g CO2
carbon monoxide: 2C(s) + O2(g) 2CO(g)
0.4163 mol C
2 mol CO
2 mol C
= 0.4163 mol CO
0.4163 mol CO
28.01 g CO
1 mol CO
= 11.7 g CO
174
Chapter 9: Chemical Quantities
30.
2NaHCO3(s) Na2CO3(s) + H2O(g) + CO2(g)
molar masses: NaHCO3, 84.01 g; Na2CO3, 106.0 g
1.52 g NaHCO3 ×
3
3
1 mol NaHCO
84.01 g NaHCO
= 0.01809 mol NaHCO3
0.01809 mol NaHCO3 ×
2
3
3
1 mol Na CO
2 mol NaHCO
= 0.009047 mol Na2CO3
0.009047 mol Na2CO3 ×
2
3
2
3
106.0 g Na CO
1 mol Na CO
= 0.959 g Na2CO3
31.
2Fe(s) + 3Cl2(g) 2FeCl3(s)
millimolar masses: iron, 55.85 mg; FeCl3, 162.2 mg
15.5 mg Fe
1 mmol Fe
55.85 mg Fe
= 0.2775 mmol Fe
0.2775 mmol Fe
3
2 mmol FeCl
2 mmol Fe
= 0.2775 mmol FeCl3
0.2775 mmol FeCl3
3
3
162.2 mg FeCl
1 mmol FeCl
= 45.0 mg FeCl3
32.
C6H12O6(aq) 2C2H5OH(aq) + 2CO2(g)
molar masses: C6H12O6, 180.2 g; C2H5OH, 46.07 g
5.25 g C6H12O6 ×
6
12
6
6
12
6
1 mol C H O
180.2 g C H O
= 0.02913 mol 6 12 6
C H O
0.02913 mol 6 12 6
C H O ×
2
5
6
12
6
2 mol C H OH
1 mol C H O
= 0.5826 mol C2H5OH
0.5286 mol C2H5OH ×
2
5
2
5
46.07 g C H OH
1 mol C H OH
= 2.68 g ethyl alcohol
33.
H2SO3(aq) H2O(l) + SO2(g)
molar masses: H2SO3, 82.09 g; SO2, 64.07 g
4.25 g H2SO3 ×
2
3
2
3
1 mol H SO
82.09 g H SO
= 0.05177 mol H2SO3
0.05177 mol H2SO3 ×
2
2
3
1 mol SO
1 mol H SO
= 0.05177 mol SO2
0.05177 mol SO2 ×
2
2
64.07 g SO
1 mol SO
= 3.32 g SO2
175
Chapter 9: Chemical Quantities
34.
The balanced equation for the reaction is:
2H2O2(aq) 2H2O(l) + O2(g)
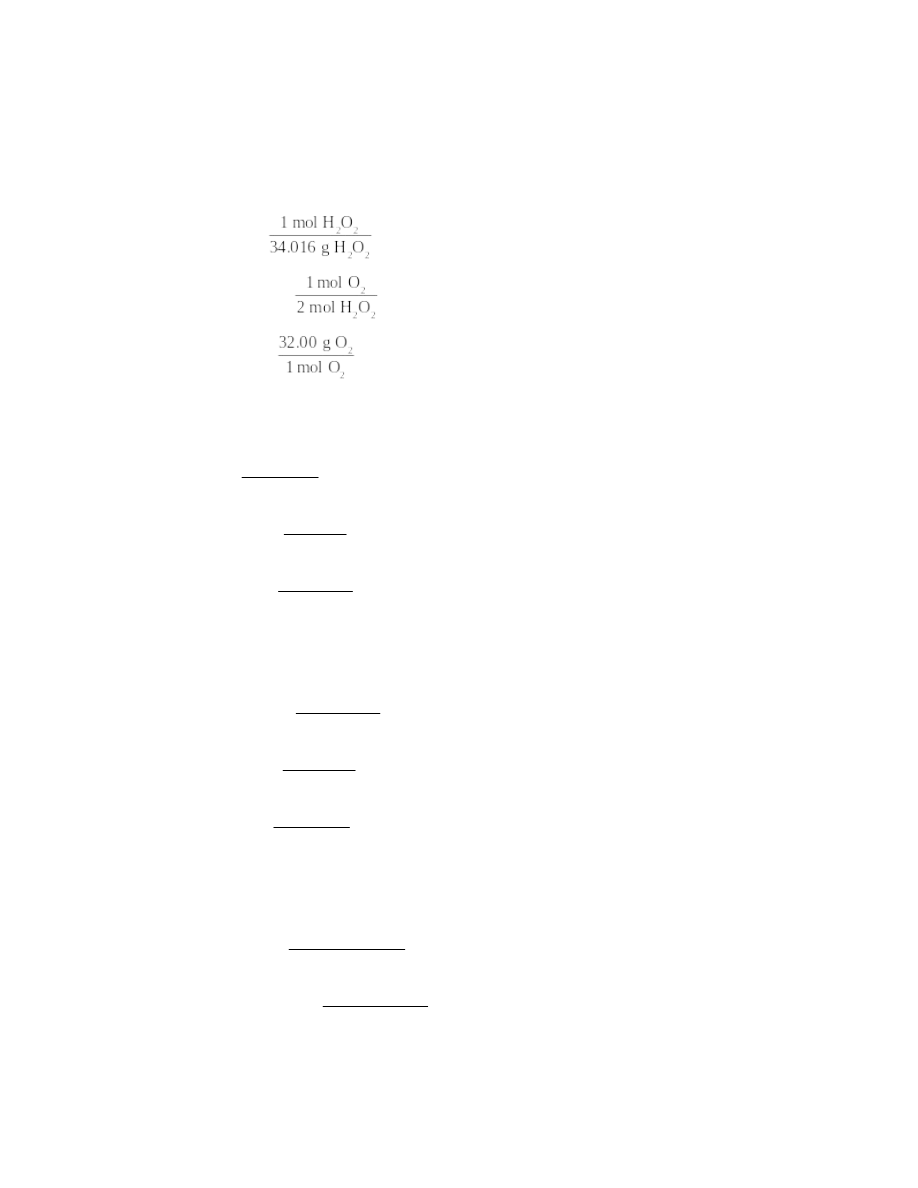
molar masses: H2O2, 34.016 g; O2, 32.00 g
10.00 g H2O2 ×
= 0.2940 mol H2O2
0.2940 mol H2O2 ×
= 0.1470 mol O2
0.1470 mol O2 ×
= 4.704 g O2
35.
P4(s) + 5O2(g) 2P2O5(s)
molar masses: P4, 123.88 g; O2, 32.00 g
4.95 g P4 ×
4
4
1 mol P
123.88 g P
= 0.03996 mol P4
0.03996 mol P4 ×
2
4
5 mol O
1 mol P
= 0.1998 mol O2
0.1998 mol O2 ×
2
2
32.00 g O
1 mol O
= 6.39 g O2
36.
4HgS(s) + 4CaO(s) 4Hg(l) + 3CaS(s) + CaSO4(s)
molar masses: HgS, 232.7 g Hg, 200.6 g; 10.0 kg = 1.00 × 104 g
1.00 × 104 g HgS ×
1 mol HgS
232.7 g HgS
= 42.97 mol HgS
42.97 mol HgS ×
4 mol Hg
4 mol HgS
= 42.97 mol Hg
42.97 mol Hg ×
200.6 g Hg
1 mol Hg
= 8.62 × 103 g Hg = 8.62 kg Hg
37.
2NH4NO3(s) 2N2(g) + O2(g) + 4H2O(g)
molar masses: NH4NO3, 80.05 g; N2, 28.02 g; 02, 32.00 g; H2O, 18.02 g
1.25 g NH4NO3
4
3
4
3
1 mol NH NO
80.05 g NH NO
= 0.0156 mol NH4NO3
0.0156 mol NH4NO3
2
4
3
2 mol N
2 mol NH NO
= 0.0156 mol N2
176
Chapter 9: Chemical Quantities
0.0156 mol N2
2
2
28.02 g N
1 mol N
= 0.437 g N2
0.0156 mol NH4NO3
2
4
3
1 mol O
2 mol NH NO
= 0.00780 mol O2
0.00780 mol O2
2
2
32.00 g O
1 mol O
= 0.250 g O2
0.0156 mol NH4NO3
2
4
3
4 mol H O
2 mol NH NO
= 0.0312 mol H2O
0.0312 mol H2O
2
2
18.02 g H O
1 mol H O
= 0.562 g H2O
As a check, note that 0.437 g + 0.250 g + 0.562 g = 1.249 g = 1.25 g.
38.
C12H22O11(s) 12C(s) + 11H2O(g)
molar masses: C12H22O11, 342.3 g; C, 12.01
1.19 g C12H22O11 ×
12
22
11
12
22
11
1 mol C H O
342.3 g C H O
= 3.476 × 10–3 mol 12 22 11
C H O
3.476 × 10–3 mol
12
22
11
C H O ×
12
22
11
12 mol C
1 mol C H O
= 0.04172 mol C
0.04172 mol C ×
12.01 g C
1 mol C
= 0.501 g C
39.
SOCl2(l) + H2O(l) SO2(g) + 2HCl(g)
molar masses: SOCl2, 119.0 g; H2O, 18.02 g
35.0 g SOCl2
2
2
1 mol SOCl
119.0 g SOCl
= 0.294 mol SOCl2
0.294 mol SOCl2
2
2
1 mol H O
1 mol SOCl
= 0.294 mol H2O
0.294 mol H2O
2
2
18.02 g H O
1 mol H O
= 5.30 g H2O
40.
The balanced equation is:
2C8H18 + 25O2 16CO2 + 18H2O
molar masses: C8H18, 114.22 g; CO2, 44.01 g
1 lb of CO2 = 453.59 g CO2
453.59 g CO2 ×
2
2
1 mol CO
44.01 g CO
= 10.31 mol CO2
From the balanced chemical equation, we can calculate the number of moles and number of
grams of pure octane that would be required to produce 10.31 mol CO2.
177

Chapter 9: Chemical Quantities
10.31 mol CO2 ×
8
18
2
2 mol C H
16 mol CO
= 1.288 mol C8H18
1.288 mol C8H18 ×
8
18
8
18
114.22 g C H
1 mol C H
= 147.2 g C8H18
From the density of C8H18 we can calculate the volume of 147.2 g C8H18.
147.2 g C8H18 ×
8
18
8
18
1 mL C H
0.75 g C H
= 196.3 mL C8H18 (2.0 × 102 mL to two significant figures)
From the preceding, we know that to travel 1 mile, we need approximately 200 mL of octane
1 mi
1000 mL 3.7854 L
196.3 mL
1 L
1 gal
= approximately 19 mi/gal
41.
To determine the limiting reactant, first calculate the number of moles of each reactant present.
Then determine how these numbers of moles correspond to the stoichiometric ratio indicated by
the balanced chemical equation for the reaction. Specific answer depends on student response.
42.
To determine the limiting reactant, first calculate the number of moles of each reactant present.
Then determine how these numbers of moles correspond to the stoichiometric ratio indicated by
the balanced chemical equation for the reaction.
43.
(c); The limiting reactant must be determined by the starting masses of each reactant, molar
masses of each reactant, and the ratio in which they react in the balanced chemical equation.
44.
(a); When checking to see if mass is conserved in a reaction, add the masses of the two reactants
before the reaction takes place. This should be equal to the total mass of products after the
reaction plus whatever excess reactant is leftover.
45.
a.
Na2B4O7(s) + H2SO4(aq) + 5H2O(l) 4H3BO3(s) + Na2SO4(aq)
molar masses: Na2B4O7, 201.2 g; H2SO4, 98.09 g; H2O, 18.02 g
5.00 g Na2B4O7
1 mol
201.2 g
= 0.0249 mol Na2B4O7
5.00 g H2SO4
1 mol
98.09 g
= 0.0510 mol H2SO4
5.00 g H2O
1 mol
18.02 g
= 0.277 mol H2O
Na2B4O7 is the limiting reactant.
mol H2SO4 remaining unreacted = 0.0510 – 0.0249 = 0.0261 mol
mol H2O remaining unreacted = 0.277 – 5(0.0249) = 0.153 mol
mass of H2SO4 remaining = 0.0261 mol
98.09 g
1 mol
= 2.56 g H2SO4
mass of H2O remaining = 0.153 mol
18.02 g
1 mol
= 2.76 g H2O
178

Chapter 9: Chemical Quantities
b.
CaC2(s) + 2H2O(l) Ca(OH)2(s) + C2H2(g)
molar masses: CaC2, 64.10 g; H2O, 18.02 g
5.00 g CaC2
1 mol
64.10 g
= 0.0780 mol CaC2
5.00 g H2O
1 mol
18.02 g
= 0.277 mol H2O
CaC2 is the limiting reactant; water is present in excess.
mol of H2O remaining = 0.277 – 2(0.0780) = 0.121 mol H2O
mass of H2O remaining = 0.121 mol
18.02 g
1 mol
= 2.18 g H2O
c.
2NaCl(s) + H2SO4(l) 2HCl(g) + Na2SO4(s)
molar masses: NaCl, 58.44 g; H2SO4, 98.09 g
5.00 g NaCl
1 mol
58.44 g
= 0.0856 mol NaCl
5.00 g H2SO4
1 mol
98.09 g
= 0.0510 mol H2SO4
NaCl is the limiting reactant; H2SO4 is present in excess.
mol H2SO4 that reacts = 0.5(0.0856) = 0.0428 mol H2SO4
mol H2SO4 remaining = 0.0510 – 0.0428 = 0.0082 mol
mass of H2SO4 remaining = 0.0082 mol
98.09 g
1 mol
= 0.80 g H2SO4
d.
SiO2(s) + 2C(s) Si(l) + 2CO(g)
molar masses: SiO2, 60.09 g; C, 12.01 g
5.00 g SiO2
1 mol
60.09 g
= 0.0832 mol SiO2
5.00 g C
1 mol
12.01 g
= 0.416 mol C
SiO2 is the limiting reactant; C is present in excess.
mol C remaining = 0.416 – 2(0.0832) = 0.250 mol
mass of C remaining = 0.250 mol
12.01 g
1 mol
= 3.00 g C
179
Chapter 9: Chemical Quantities
46.
a.
S(s) + 2H2SO4(aq) 3SO2(g) + 2H2O(l)
Molar masses: S, 32.07 g; H2SO4, 98.09 g; SO2, 64.07 g; H2O, 18.02 g
5.00 g S
1 mol
32.07 g
= 0.1559 mol S
5.00 g H2SO4
1 mol
98.09 g
= 0.05097 mol H2SO4
According to the balanced chemical equation, we would need twice as much sulfuric acid
as sulfur for complete reaction of both reactants. We clearly have much less sulfuric acid
present than sulfur: sulfuric acid is the limiting reactant. The calculation of the masses of
products produced is based on the number of moles of the sulfuric acid.
0.05097 mol H2SO4
2
2
2
4
2
3 mol SO
64.07 g SO
2 mol H SO
1 mol SO
= 4.90 g SO2
0.05097 mol H2SO4
2
2
2
4
2
2 mol H O
18.02 g H O
2 mol H SO
1 mol H O
= 0.918 g H2O
b.
MnO2(s) + 2H2SO4(aq) Mn(SO4)2 + 2H2O(l)
molar masses: MnO2, 86.94 g; H2SO4 98.09 g; Mn(SO4)2, 247.1 g; H2O, 18.02 g
5.00 g MnO2
1 mol
86.94 g
= 0.05751 mol MnO2
5.00 g H2SO4
1 mol
98.09 g
= 0.05097 mol H2SO4
According to the balanced chemical equation, we would need twice as much sulfuric acid
as manganese(IV) oxide for complete reaction of both reactants. We do not have this
much sulfuric acid, so sulfuric acid must be the limiting reactant. The amount of each
product produced will be based on the sulfuric acid reacting completely.
0.05097 mol H2SO4
4 2
4 2
4 2
1 mol Mn(SO )
247.1 g Mn(SO )
2 mol H2SO4
1 mol Mn(SO )
= 6.30 g Mn(SO4)2
0.05097 mol H2SO4
2
2
2
4
2
2 mol H O
18.02 g H O
2 mol H SO
1 mol H O
= 0.918 g H2O
c.
2H2S(g) + 3O2(g) 2SO2(g) + 2H2O(l)
Molar masses: H2S, 34.09 g; O2, 32.00 g; SO2, 64.07 g; H2O, 18.02 g
5.00 g H2S
1 mol
34.09 g
= 0.1467 mol H2S
5.00 g O2
1 mol
32.00 g
= 0.1563 mol O2
180

Chapter 9: Chemical Quantities
According to the balanced equation, we would need 1.5 times as much O2 as H2S for
complete reaction of both reactants. We don’t have that much O2, so O2 must be the
limiting reactant that will control the masses of each product produced.
0.1563 mol O2
2
2
2
2
2 mol SO
64.07 g SO
3 mol O
1 mol SO
= 6.67 g SO2
0.1563 mol O2
2
2
2
2
2 mol H O 18.02 g H O
3 mol O
1 mol H O
= 1.88 g H2O
d.
3AgNO3(aq) + Al(s) 3Ag(s) + Al(NO3)3(aq)
Molar masses: AgNO3, 169.9 g; Al, 26.98 g; Ag, 107.9 g; Al(NO3)3, 213.0 g
5.00 g AgNO3
1 mol
169.9 g
= 0.02943 mol AgNO3
5.00 g Al
1 mol
26.98 g
= 0.1853 mol Al
According to the balanced chemical equation, we would need three moles of AgNO3 for
every mole of Al for complete reaction of both reactants. We in fact have fewer moles of
AgNO3 than aluminum, so AgNO3 must be the limiting reactant. The amount of product
produced is calculated from the number of moles of the limiting reactant present:
0.02943 mol AgNO3
3
3 mol Ag
107.9 g Ag
3 mol AgNO
1 mol Ag
= 3.18 g Ag
0.02943 mol AgNO3
3 3
3 3
3
3 3
1 mol Al(NO )
213.0 g Al(NO )
3 mol AgNO
1 mol Al(NO )
= 2.09 g
47.
Before any calculations are attempted, the equations must be balanced.
a.
C3H8(g) + 5O2(g) 3CO2(g) + 4H2O(g)
molar masses: C3H8, 44.09 g; O2, 32.00 g; CO2, 44.01 g; H2O, 18.02 g
10.0 g C3H8
1 mol
44.09 g
= 0.2268 mol C3H8
10.0 g O2
1 mol
32.00 g
= 0.3125 mol O2
For 0.2268 mol C3H8, the amount of O2 that would be needed is
0.2268 mol C3H8
2
3
8
5 mol O
1 mol C H
= 1.134 mol O2
Because we do not have this amount of O2, then O2 is the limiting reactant.
0.3125 mol O2
2
2
2
2
3 mol CO
44.01 g CO
5 mol O
1 mol CO
= 8.25 g CO2
181

Chapter 9: Chemical Quantities
0.3125 mol O2
2
2
2
2
4 mol H O 18.02 g H O
5 mol O
1 mol H O
= 4.51 g H2O
b.
2Al(s) + 3Cl2(g) 2AlCl3(s)
molar masses: Al, 26.98 g; Cl2, 70.90 g; AlCl3, 133.3 g
10.0 g Al
1 mol
26.98 g
= 0.3706 mol Al
10.0 g Cl2
1 mol
70.90 g
= 0.1410 mol Cl2
For 0.1410 mol Cl2(g), the amount of Al(s) required is
0.1410 mol Cl2
2
2 mol Al
3 mol Cl
= 0.09400 mol Al
We have far more than this amount of Al(s) present, so Cl2(g) must be the limiting
reactant that will control the amount of AlCl3 which forms.
0.1410 mol Cl2
3
3
2
3
2 mol AlCl
133.3 g AlCl
3 mol Cl
1 mol AlCl
= 12.5 g AlCl3
c.
2NaOH(s) + CO2(g) Na2CO3(s) + H2O(l)
molar masses: NaOH, 40.00 g; CO2, 44.01 g; Na2CO3, 106.0 g; H2O, 18.02 g
10.0 g NaOH
1 mol
40.00 g
= 0.2500 mol NaOH
10.0 g CO2
1 mol
44.01 g
= 0.2272 mol CO2
Without having to calculate, according to the balanced chemical equation, we would need
twice as many moles of NaOH as CO2 for complete reaction. For the amounts calculated
above, there is not nearly enough NaOH present for the amount of Cl2 used: NaOH is the
limiting reactant.
0.2500 mol NaOH
2
3
2
3
2
3
1 mol Na CO
106.0 g Na CO
2 mol NaOH
1 mol Na CO
= 13.3 g Na2CO3
0.2500 mol NaOH
2
2
2
1 mol H O
18.02 g H O
2 mol NaOH
1 mol H O
= 2.25 g H2O
d.
NaHCO3(s) + HCl(aq) NaCl(aq) + H2O(l) + CO2(g)
molar masses: NaHCO3, 84.01 g; HCl, 36.46 g; NaCl, 58.44 g; H2O, 18.02 g; CO2, 44.01
g
10.0 g NaHCO3
1 mol
84.01 g
= 0.1190 mol NaHCO3
10.0 g HCl
1 mol
36.46 g
= 0.2742 mol HCl
182

Chapter 9: Chemical Quantities
Because the coefficients of NaHCO3(s) and HCl(aq) are both one in the balanced
chemical equation for the reaction, there is not enough NaHCO3 present to react with the
amount of HCl present: the 0.1190 mol NaHCO3 present is the limiting reactant. Because
all the coefficients of the products are also each one, then if 0.1190 mol NaHCO3 reacts
completely (with 0.1190 mol HCl), 0.1190 mol of each product will form.
0.1190 mol NaCl
58.44 g
1 mol
= 6.95 g NaCl
0.1190 mol H2O
18.02 g
1 mol
= 2.14 g H2O
0.1190 mol CO2
44.01 g
1 mol
= 5.24 g CO2
48.
a.
CS2(l) + 3O2(g) CO2(g) + 2SO2(g)
Molar masses: CS2, 76.15 g; O2, 32.00 g; CO2, 44.01 g
1.00 g CS2
1 mol
76.15 g
= 0.01313 mol CS2
1.00 g O2
1 mol
32.00 g
= 0.03125 mol O2
From the balanced chemical equation, we would need three times as much oxygen as
carbon disulfide for complete reaction of both reactants. We do not have this much
oxygen, and so oxygen must be the limiting reactant.
0.03125 mol O2
2
2
2
2
1 mol CO
44.01 g CO
3 mol O
1 mol CO
= 0.458 g CO2
b.
2NH3(g) + CO2(g) CN2H4O(s) + H2O(l)
Molar masses: NH3, 17.03 g; CO2, 44.01 g; H2O, 18.02 g
1.00 g NH3
1 mol
17.03 g
= 0.05872 mol NH3
1.00 g CO2
1 mol
44.01 g
= 0.02272 mol CO2
The balanced chemical equation tells us that we would need twice as many moles of
ammonia as carbon dioxide for complete reaction of both reactants. We have more than
this amount of ammonia present, so the reaction will be limited by the amount of carbon
dioxide present.
0.02272 mol CO2
2
2
2
2
1 mol H O 18.02 g H O
1 mol CO
1 mol H O
= 0.409 g H2O
c.
H2(g) + MnO2(s) MnO(s) + H2O(l)
Molar masses: H2, 2.016 g; MnO2, 86.94 g; H2O, 18.02 g
183
Chapter 9: Chemical Quantities
1.00 g H2
1 mol
2.016 g
= 0.496 mol H2
1.00 g MnO2
1 mol
86.94 g
= 0.0115 mol MnO2
Because the coefficients of both reactants in the balanced chemical equation are the same,
we would need equal amounts of both reactants for complete reaction. Therefore,
manganese(IV) oxide must be the limiting reactant and controls the amount of product
obtained.
0.0115 mol MnO2
2
2
2
2
1 mol H O
18.02 g H O
1 mol MnO
1 mol H O
= 0.207 g H2O
d.
I2(s) + Cl2(g) 2ICl(g)
Molar masses: I2, 253.8 g; Cl2, 70.90 g; ICl, 162.35 g
1.00 g I2
1 mol
253.8 g
= 0.00394 mol I2
1.00 g Cl2
1 mol
70.90 g
= 0.0141 mol Cl2
From the balanced chemical equation, we would need equal amounts of I2 and Cl2 for
complete reaction of both reactants. As we have much less iodine than chlorine, iodine
must be the limiting reactant.
0.00394 mol I2
2
2 mol ICl 162.35 g ICl
1 mol I
1 mol ICl
= 1.28 g ICl
49.
a.
UO2(s) + 4HF(aq) UF4(aq) + 2H2O(l)
UO2 is the limiting reactant; 1.16 g UF4, 0.133 g H2O
b.
2NaNO3(aq) + H2SO4(aq) Na2SO4(aq) + 2HNO3(aq)
NaNO3 is the limiting reactant; 0.836 g Na2SO4; 0.741 g HNO3
c.
Zn(s) + 2HCl(aq) ZnCl2(aq) + H2(g)
HCl is the limiting reactant; 1.87 g ZnCl2; 0.0276 g H2
d.
B(OH)3(s) + 3CH3OH(l) B(OCH3)3(s) + 3H2O(l)
CH3OH is the limiting reactant; 1.08 g B(OCH3)3; 0.562 g H2O
50.
a.
2Al(s) + 6HCl(aq) 2AlCl3(aq) + 3H2(g)
HCl is the limiting reactant; 18.3 g AlCl3; 0.415 g H2
b.
2NaOH(aq) + CO2(g) Na2CO3(aq) + H2O(l)
NaOH is the limiting reactant; 19.9 g Na2CO3; 3.38 g H2O
c.
Pb(NO3)2(aq) + 2HCl(aq) PbCl2(s) + 2HNO3(aq)
Pb(NO3)2 is the limiting reactant; 12.6 g PbCl2; 5.71 g HNO3
184

Chapter 9: Chemical Quantities
d.
2K(s) + I2(s) 2KI(s)
I2 is the limiting reactant; 19.6 g KI
51.
Pb(C2H3O2)2(aq) + H2O(l) + CO2(g) PbCO3(s) + 2HC2H3O2(aq)
molar masses: Pb(C2H3O2)2, 325.3 g; CO2, 44.01 g; PbCO3, 267.21 g
1.25 g Pb(C2H3O2)2 ×
1 mol
325.3 g
= 0.00384 mol Pb(C2H3O2)2
5.95 g CO2 ×
1 mol
44.01 g
= 0.135 mol CO2
Pb(C2H3O2)2 is the limiting reactant, which determines the yield of product.
0.00384 mol Pb(C2H3O2)2 ×
3
3
2
3
2
3
2
1 mol PbCO
267.21 g PbCO
1 mol Pb C H O
1 mol PbCO
= 1.03 g PbCO3
52.
CuO(s) + H2SO4(aq) CuSO4(aq) + H2O(l)
molar masses: CuO, 79.55 g; H2SO4, 98.09 g
2.49 g CuO
1 mol CuO
79.55 g CuO
= 0.0313 mol CuO
5.05 g H2SO4
2
4
2
4
1 mol H SO
98.09 g H SO
= 0.0515 mol H2SO4
Since the reaction is of 1:1 stoichiometry, CuO must be the limiting reactant since it is present in
the lesser amount on a molar basis.
53.
PbO(s) + C(s) Pb(l) + CO(g)
molar masses: PbO, 223.2 g; C, 12.01 g; Pb, 207.2 g
50.0 103 g PbO
1 mol
223.2 g
= 224.0 mol PbO
50.0 103 g C
1 mol
12.01 g
= 4163 mol C
PbO is the limiting reactant.
224.0 mol PbO
1 mol Pb
207.2 g Pb
1 mol PbO
1 mol Pb
= 4.64 104 g = 46.4 kg Pb
54.
4Fe(s) + 3O2(g) 2Fe2O3(s)
Molar masses: Fe, 55.85 g; Fe2O3, 159.7 g
1.25 g Fe
1 mol
55.85 g
= 0.0224 mol Fe present
Calculate how many mol of O2 are required to react with this amount of Fe
185

Chapter 9: Chemical Quantities
0.0224 mol Fe
2
3 mol O
4 mol Fe
= 0.0168 mol O2
Because we have more O2 than this, Fe must be the limiting reactant.
0.0224 mol Fe
2
3
2
3
2
3
2 mol Fe O
159.7 g Fe O
4 mol Fe
1 mol Fe O
= 1.79 g Fe2O3
55.
Ag+(aq) + Cl–(aq) AgCl(s)
molar masses: AgNO3, 169.91 g; NaCl, 58.44 g
The number of moles of silver ion present will be the same as the number of moles of silver
nitrate taken because each formula unit of silver nitrate contains one silver ion.
1.15 g AgNO3
3
3
1 mol AgNO
169.91 g AgNO
= 0.00677 mol AgNO3 = 0.00677 mol Ag+ ion
The number of moles of chloride ion present will be the same as the number of moles of sodium
chloride taken, because each formula unit of sodium chloride contains one sodium ion.
5.45 g NaCl
1 mol NaCl
58.44 g NaCl
= 0.0933 mol NaCl = 0.0933 mol Cl– ion
Because the balanced chemical equation indicates a 1:1 stoichiometry for the reaction, there is not
nearly enough silver ion present (0.00677 mol) to precipitate the amount of chloride ion in the
sample (0.0933 mol).
56.
CaCl2(aq) + Na2SO4(aq) CaSO4(s) + 2NaCl(aq)
molar masses: CaCl2, 110.98 g; Na2SO4, 142.05 g
5.21 g CaCl2
2
2
1 mol CaCl
110.98 g CaCl
= 0.0469 mol CaCl2 = 0.0469 mol Ca2+ ion
4.95 g Na2SO4
2
4
2
4
1 mol Na SO
142.05 g Na SO
= 0.0348 mol Na2SO4 = 0.0348 mol SO42– ion
Because the balanced chemical equation indicates a 1:1 stoichiometry for the reaction, there is not
nearly enough sulfate ion present (0.0348 mol) to precipitate the amount of calcium ion in the
sample (0.0469 mol). Sodium sulfate (sulfate ion) is the limiting reactant. Calcium chloride
(calcium ion) is present in excess.
57.
BaO2(s) + 2HCl(aq) H2O2(aq) + BaCl2(aq)
molar masses: BaO2, 169.3 g; HCl, 36.46 g; H2O2, 34.02 g
1.50 g BaO2
1 mol
169.3 g
= 8.860 10–3 mol BaO2
25.0 mL solution
0.0272 g HCl
1 mL solution
= 0.680 g HCl
0.680 g HCl
1 mol
36.46 g
= 1.865 10–2 mol HCl
186

Chapter 9: Chemical Quantities
BaO2 is the limiting reactant.
8.860 10–3 mol BaO2
2
2
2
2
2
2
1 mol H O
34.02 g H O
1 mol BaO2
1 mol H O
= 0.301 g H2O2
58.
SiO2(s) + 3C(s) 2CO(g) + SiC(s)
molar masses: SiO2, 60.09 g; SiC, 40.10 g; 1.0 kg = 1.0 103 g
1.0 103 g SiO2
1 mol
60.09 g
= 16.64 mol SiO2
From the balanced chemical equation, if 16.64 mol of SiO2 were to react completely (an excess of
carbon is present), then 16.64 mol of SiC should be produced (the coefficients of SiO2 and SiC
are the same).
16.64 mol SiC
40.01 g
1 mol
= 6.7 102 g SiC = 0.67 kg SiC
59.
The theoretical yield represents the yield we calculate from the stoichiometry of the reaction and
the masses of reactants taken for the experiment. The actual yield is what is actually obtained in
an experiment. The percent yield is the ratio of what is actually obtained to the theoretical amount
that could be obtained, converted to a percent basis.
60.
If the reaction is performed in a solvent, the product may have a substantial solubility in the
solvent; the reaction may come to equilibrium before the full yield of product is achieved (see
Chapter 16); loss of product may occur through operator error.
61.
Percent yield =
actual yield
theoretical yield
100 =
1.23 g
1.44 g
100 = 85.4%
62.
2NaN3(s) 2Na(s) + 3N2(g)
molar mass: NaN3, 65.02 g; Na, 22.99 g
10.5 g NaN3 ×
= 0.161 mol NaN3
0.161 mol NaN3 ×
= 0.161 mol Na
0.161 mol Na ×
= 3.71 g Na theoretical yield
% yield =
× 100 = 76.5%
63.
S8(s) + 8Na2SO3(aq) + 40H2O(l) 8Na2S2O3 5H2O
molar masses: S8, 256.6 g; Na2SO3, 126.1 g; Na2S2O3 5H2O, 248.2 g
3.25 g S8
1 mol
256.6 g
= 0.01267 mol S8
187
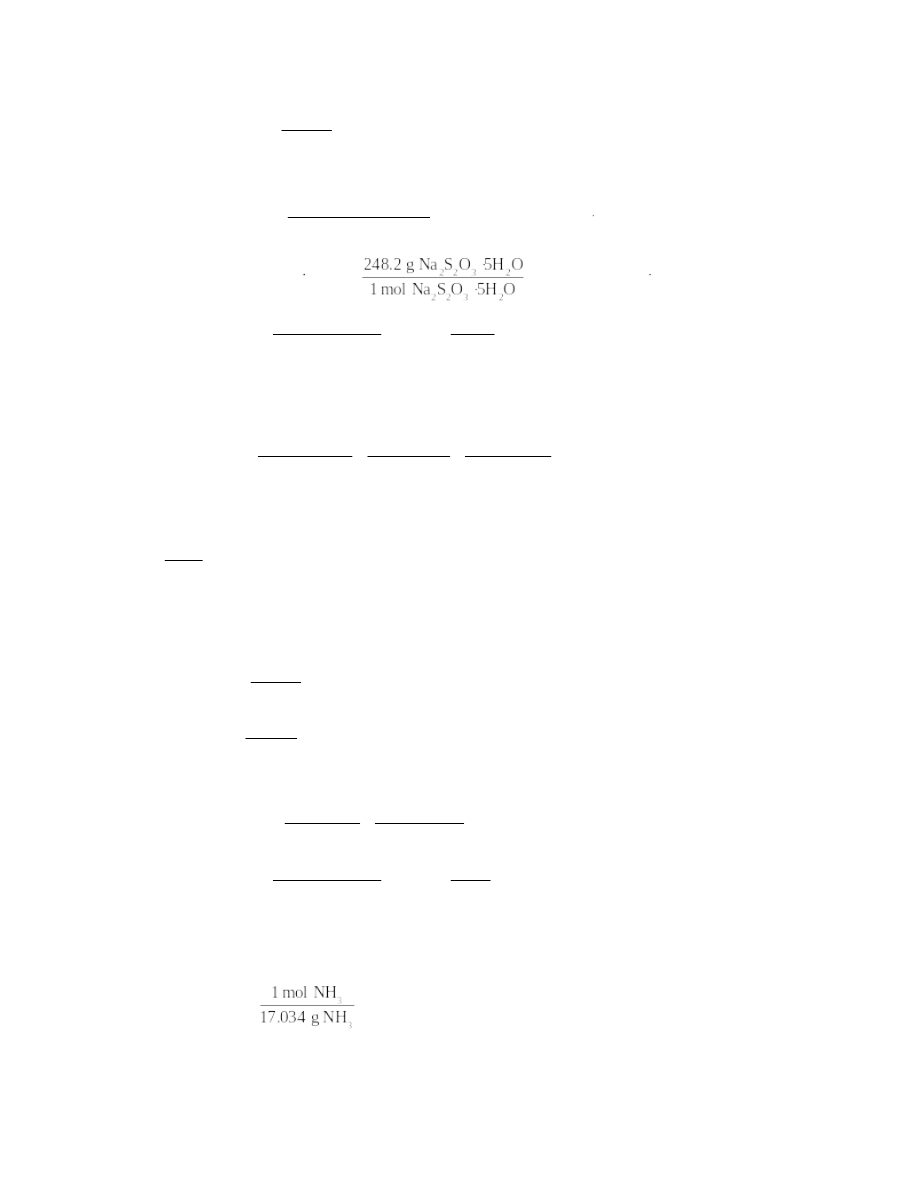
Chapter 9: Chemical Quantities
13.1 g Na2SO3
1 mol
126.1 g
= 0.1039 mol Na2SO3
S8 is the limiting reactant.
0.01267 mol S8
.
2 2
3
2
8
8 mol Na S O 5H O
1 mol S
= 0.1014 mol Na2S2O3 5H2O
0.1014 mol Na2S2O3 5H2O
= 25.2 g Na2S2O3 5H2O
Percent yield =
actual yield
theoretical yield
100 =
5.26 g
25.2 g
100 = 20.9%
64.
2LiOH(s) + CO2(g) Li2CO3(s) + H2O(g)
molar masses: LiOH, 23.95 g; CO2, 44.01 g
155 g LiOH ×
2
2
2
1 mol CO
44.01 g CO
1 mol LiOH
23.95 g LiOH 2 mol LiOH
1 mol CO
= 142 g CO2
As the cartridge has only absorbed 102 g CO2 out of a total capacity of 142 g CO2, the cartridge
has absorbed
102 g
142 g
100 = 71.8% of its capacity.
65.
Xe(g) + 2F2(g) XeF4(s)
molar masses: Xe, 131.3 g; F2, 38.00 g; XeF4, 207.3 g
130. g Xe
1 mol
131.3 g
= 0.9901 mol Xe
100. g F2
1 mol
38.00 g
= 2.632 mol F2
Xe is the limiting reactant.
0.9901 mol Xe
4
4
4
1 mol XeF
207.3 g XeF
1 mol Xe
1 mol XeF
= 205 g XeF4
Percent yield =
actual yield
theoretical yield
100 =
145 g
205 g
100 = 70.7 % of theory
66.
2NH3(g) + 3CuO(s) N2(g) + 3Cu(s) + 3H2O(g)
molar masses: NH3, 17.034 g; CuO, 79.55 g; Cu, 63.55 g
18.1 g NH3
= 1.06 mol NH3
188
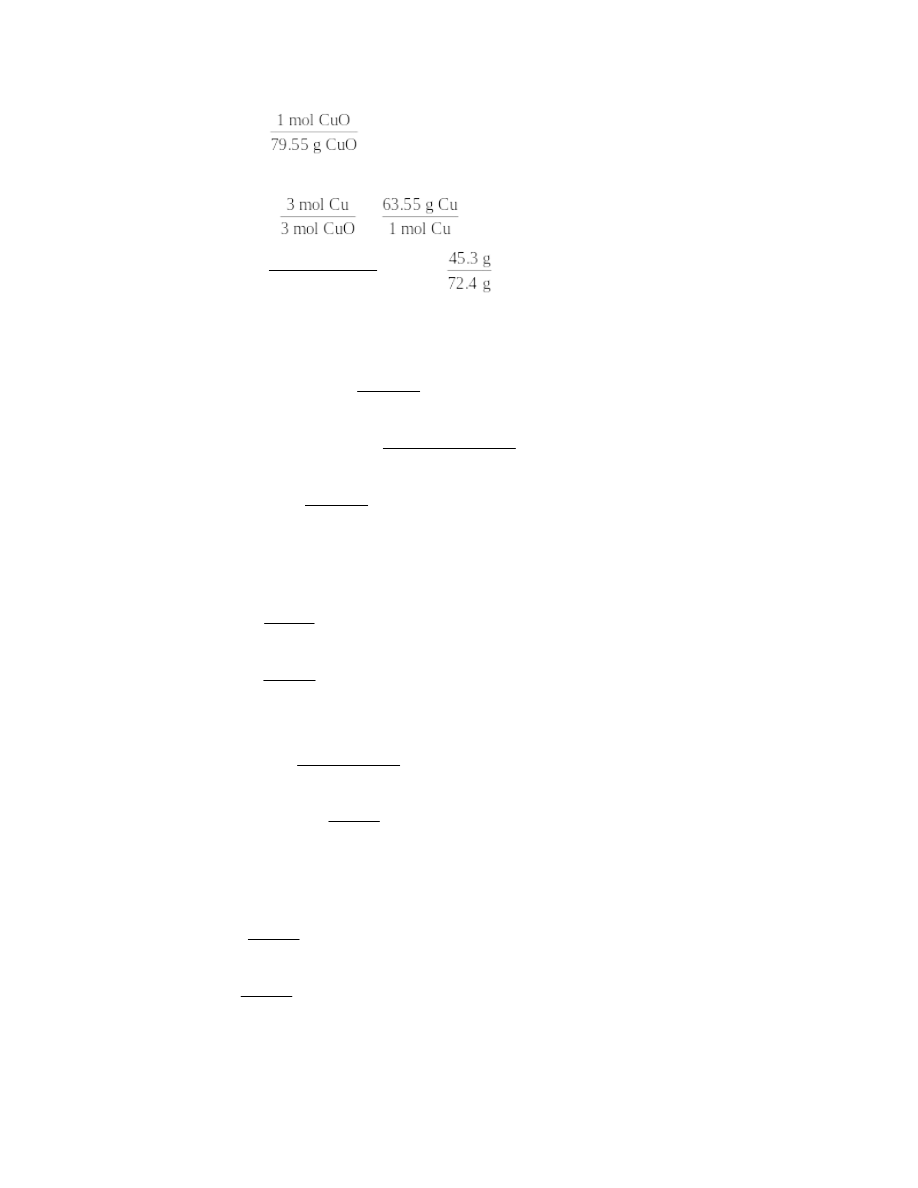
Chapter 9: Chemical Quantities
90.4 g BaCl2
= 1.14 mol CuO
CuO is the limiting reactant.
1.14 mol CuO
= 72.4 g Cu
Percent yield =
actual yield
theoretical yield
100 =
100 = 62.6%
67.
Ca(HCO3)2(aq) CaCO3(s) + CO2(g) + H2O(l)
millimolar masses: Ca(HCO3)2, 162.1 mg; CaCO3, 100.1 mg
2.0 10–3 mg Ca(HCO3)2
1 mmol
162.1 mg
= 1.23 10–5 mmol Ca(HCO3)2
1.23 10–5 mmol Ca(HCO3)2
3
3 2
1 mmol CaCO
1 mmol Ca(HCO )
= 1.23 10–5 mmol CaCO3
1.23 10–5 mmol
100.1 mg
1 mmol
= 1.2 10–3 mg = 1.2 10–6 g CaCO3
68.
NaCl(aq) + NH3(aq) + H2O(l) + CO2(s) NH4Cl(aq) + NaHCO3(s)
molar masses: NH3, 17.03 g; CO2, 44.01 g; NaHCO3, 84.01 g
10.0 g NH3
1 mol
17.03 g
= 0.5872 mol NH3
15.0 g CO2
1 mol
44.01 g
= 0.3408 mol CO2
CO2 is the limiting reactant.
0.3408 mol CO2
3
2
1 mol NaHCO
1 mol CO
= 0.3408 mol NaHCO3
0.3408 mol NaHCO3
84.01 g
1 mol
= 28.6 g NaHCO3
69.
Fe(s) + S(s) FeS(s)
molar masses: Fe, 55.85 g; S, 32.07 g; FeS, 87.92 g
5.25 g Fe
1 mol
55.85 g
= 0.0940 mol Fe
12.7 g S
1 mol
32.07 g
= 0.396 mol S
Fe is the limiting reactant.
189

Chapter 9: Chemical Quantities
0.0940 mol Fe
1 mol FeS 87.92 g FeS
1 mol Fe
1 mol FeS
= 8.26 g FeS produced
70.
C6H12O6(s) + 6O2(g) 6CO2(g) + 6H2O(g)
molar masses: glucose, 180.2 g; CO2, 44.01 g
1.00 g glucose = 5.549 10–3 mol glucose
5.549 10–3 mol glucose
2
6 mol CO
1 mol glucose
= 3.33 10–2 mol CO2
3.33 10–2 mol CO2
44.01 g
1 mol
= 1.47 g CO2
71.
Cu(s) + S(s) CuS(s)
molar masses: Cu, 63.55 g; S, 32.07 g; CuS, 95.62 g
31.8 g Cu
1 mol
63.55 g
= 0.5004 mol Cu
50.0 g S
1 mol
32.07 g
= 1.559 mol S
Cu is the limiting reactant.
0.5004 mol Cu
1 mol CuS
1 mol Cu
= 0.5004 mol CuS
0.5004 mol CuS
95.62 g
1 mol
= 47.8 g CuS
% yield =
40.0 g
47.8 g
100 = 83.7%
72.
Ba2+(aq) + SO42–(aq) BaSO4(s)
millimolar ionic masses: Ba2+, 137.3 mg; SO42–, 96.07 mg; BaCl2, 208.2 mg
150 mg SO42–
1 mmol
96.07 mg
= 1.56 millimol SO42–
As barium ion and sulfate ion react on a 1:1 stoichiometric basis, then 1.56 millimol of barium
ion is needed, which corresponds to 1.56 millimol of BaCl2
1.56 millimol BaCl2
208.2 mg
1 mmol
= 325 milligrams BaCl2 needed
73.
mass of Cl– present = 1.054 g sample
10.3 g Cl
100.0 g sample
= 0.1086 g Cl–
molar masses: Cl–, 35.45 g; AgNO3, 169.9 g; AgCl, 143.4 g
190
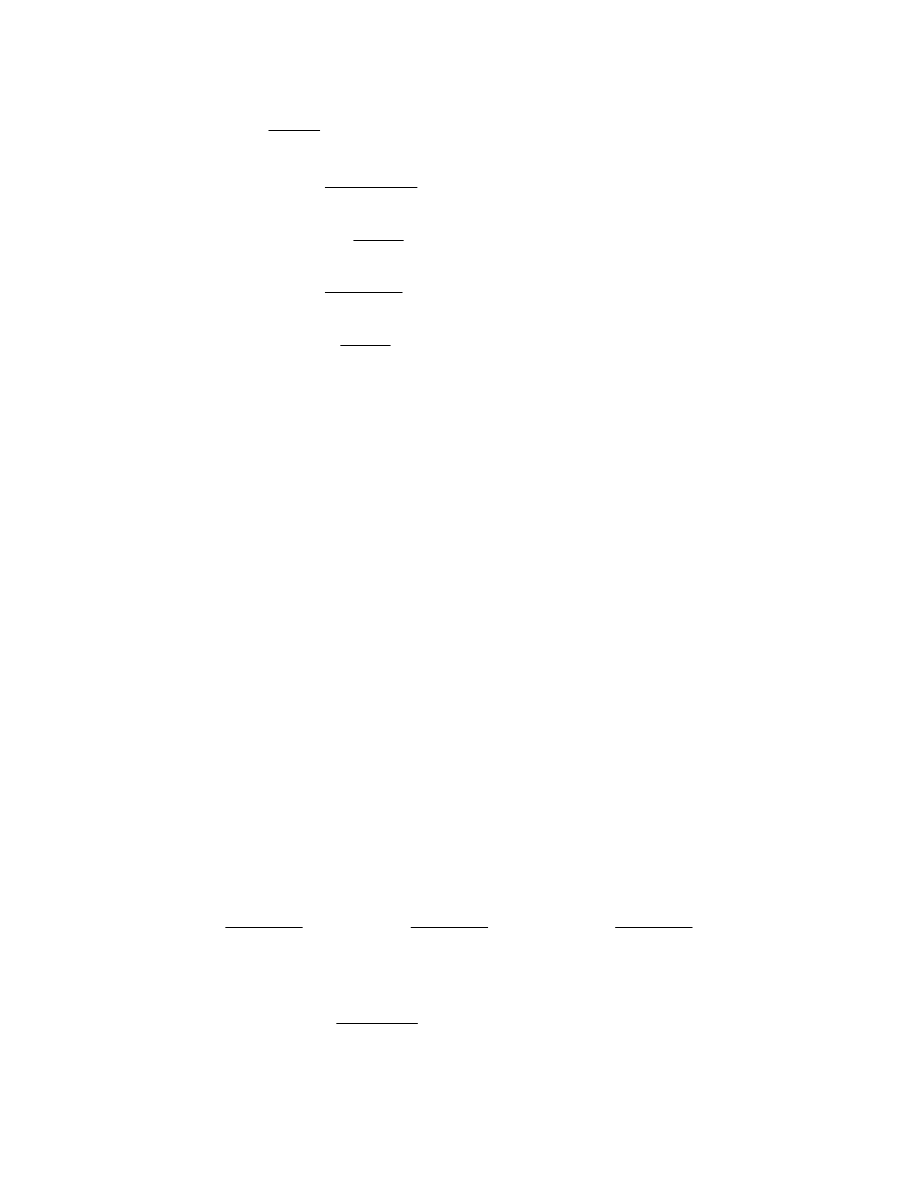
Chapter 9: Chemical Quantities
0.1086 g Cl–
1 mol
35.45 g
= 3.063 10–3 mol Cl–
3.063 10–3 mol Cl–
3
1 mol AgNO
1 mol Cl
= 3.063 10–3 mol AgNO3
3.063 10–3 mol AgNO3
169.9 g
1 mol
= 0.520 g AgNO3 required
3.063 10–3 mol Cl–
1 mol AgCl
1 mol Cl
= 3.063 10–3 mol AgCl
3.063 10–3 mol AgCl
143.4 g
1 mol
= 0.439 g AgCl produced
74.
a.
UO2(s) + 4HF(aq) UF4(aq) + 2H2O(l)
One molecule (formula unit) of uranium(IV) oxide will combine with four molecules of
hydrofluoric acid, producing one uranium(IV) fluoride molecule and two water
molecules. One mole of uranium(IV) oxide will combine with four moles of hydrofluoric
acid to produce one mole of uranium(IV) fluoride and two moles of water.
b.
2NaC2H3O2(aq) + H2SO4(aq) Na2SO4(aq) + 2HC2H3O2(aq)
Two molecules (formula units) of sodium acetate react exactly with one molecule of
sulfuric acid, producing one molecule (formula unit) of sodium sulfate and two molecules
of acetic acid. Two moles of sodium acetate will combine with one mole of sulfuric acid,
producing one mole of sodium sulfate and two moles of acetic acid.
c.
Mg(s) + 2HCl(aq) MgCl2(aq) + H2(g)
One magnesium atom will react with two hydrochloric acid molecules (formula units) to
produce one molecule (formula unit) of magnesium chloride and one molecule of
hydrogen gas. One mole of magnesium will combine with two moles of hydrochloric
acid, producing one mole of magnesium chloride and one mole of gaseous hydrogen.
d.
B2O3(s) + 3H2O(l) 2B(OH)3(aq)
One molecule of diboron trioxide will react exactly with three molecules of water,
producing two molecules of boron trihydroxide (boric acid). One mole of diboron
trioxide will combine with three moles of water to produce two moles of boron
trihydroxide (boric acid).
75.
False. For 0.40 mol of Mg(OH)2 to react, 0.80 mol of HCl will be needed. According to the
balanced equation, for a given amount of Mg(OH)2, twice as many moles of HCl is needed.
76.
For O2:
2
3
8
5 mol O
1 mol C H
For CO2:
2
3
8
3 mol CO
1 mol C H
For H2O:
2
3
8
4 mol H O
1 mol C H
77.
a.
2H2O2(l) 2H2O(l) + O2(g)
0.50 mol H2O2
2
2
2
2 mol H O
2 mol H O
= 0.50 mol H2O
191
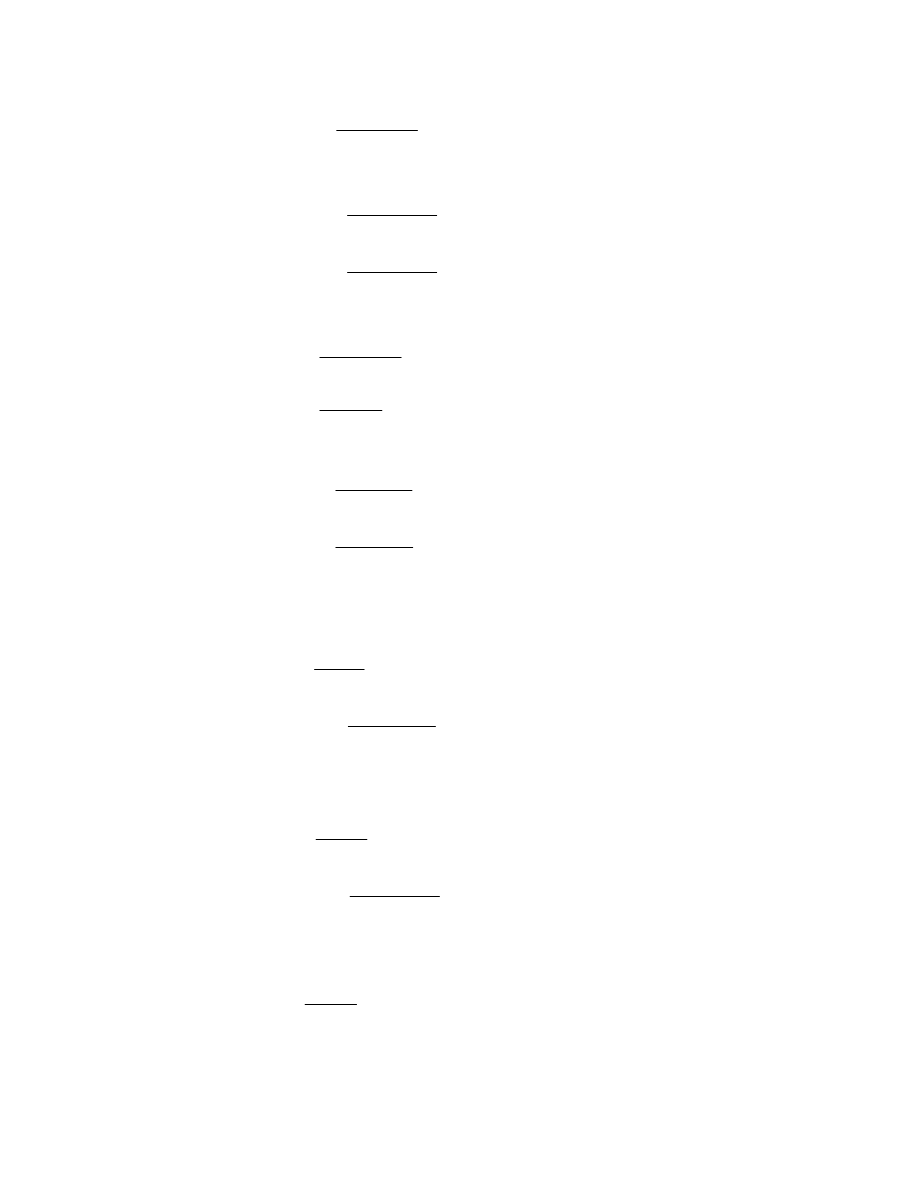
Chapter 9: Chemical Quantities
0.50 mol H2O2
2
2
2
1 mol O
2 mol H O
= 0.25 mol O2
b.
2KClO3(s) 2KCl(s) + 3O2(g)
0.50 mol KClO3
3
2 mol KCl
2 mol KClO
= 0.50 mol KCl
0.50 mol KClO3
2
3
3 mol O
2 mol KClO
= 0.75 mol O2
c.
2Al(s) + 6HCl(aq) 2AlCl3(aq) + 3H2(g)
0.50 mol Al
3
2 mol AlCl
2 mol Al
= 0.50 mol AlCl3
0.50 mol Al
2
3 mol H
2 mol Al
= 0.75 mol H2
d.
C3H8(g) + 5O2(g) 3CO2(g) + 4H2O(l)
0.50 mol C3H8
2
3
8
3 mol CO
1 mol C H
= 1.5 mol CO2
0.50 mol C3H8
2
3
8
4 mol H O
1 mol C H
= 2.0 mol H2O
78.
a.
NH3(g) + HCl(g) NH4Cl(s)
molar mass of NH3 = 17.01 g
1.00 g NH3
1 mol
17.01 g
= 0.0588 mol NH3
0.0588 mol NH3
4
3
1 mol NH Cl
1 mol NH
= 0.0588 mol NH4Cl
b.
CaO(s) + CO2(g) CaCO3(s)
molar mass CaO = 56.08 g
1.00 g CaO
1 mol
56.08 g
= 0.0178 mol CaO
0.0178 mol CaO
3
1 mol CaCO
1 mol CaO
= 0.0178 mol CaCO3
c.
4Na(s) + O2(g) 2Na2O(s)
molar mass Na = 22.99 g
1.00 g Na
1 mol
22.99 g
= 0.0435 mol Na
192

Chapter 9: Chemical Quantities
0.0435 mol Na
2
2 mol Na O
4 mol Na
= 0.0217 mol Na2O
d.
2P(s) + 3Cl2(g) 2PCl3(l)
molar mass P = 30.97 g
1.00 g P
1 mol
30.97 g
= 0.0323 mol P
0.0323 mol P
3
2 mol PCl
2 mol P
= 0.0323 mol PCl3
79.
a.
molar mass CuSO4 = 159.6 g
4.21 g CuSO4
1 mol
159.6 g
= 0.0264 mol CuSO4
b.
molar mass Ba(NO3)2 = 261.3 g
7.94 g Ba(NO3)2
1 mol
261.3 g
= 0.0304 mol Ba(NO3)2
c.
molar mass water = 18.02 g; 1.24 mg = 0.00124 g
0.00124 g
1 mol
18.02 g
= 6.88 10–5 mol H2O
d.
molar mass W = 183.9 g
9.79 g W
1 mol
183.9 g
= 5.32 10–2 mol W
e.
molar mass S = 32.07 g; 1.45 lb = 1.45(454) = 658 g
658 g S
1 mol
32.07 g
= 20.5 mol S
f.
molar mass C2H5OH = 46.07 g
4.65 g C2H5OH
1 mol
46.07 g
= 0.101 mol C2H5OH
g.
molar mass C = 12.01 g
12.01 g C
1 mol
12.01 g
= 1.00 mol C
80.
a.
molar mass HNO3 = 63.0 g
5.0 mol HNO3
63.0 g
1 mol
= 3.2 102 g HNO3
b.
molar mass Hg = 200.6 g
193
Chapter 9: Chemical Quantities
0.000305 mol Hg
200.6 g
1 mol
= 0.0612 g Hg
c.
molar mass K2CrO4 = 194.2 g
2.31 10–5 mol K2CrO4
194.2 g
1 mol
= 4.49 10–3 g K2CrO4
d.
molar mass AlCl3 = 133.3 g
10.5 mol AlCl3
133.3 g
1 mol
= 1.40 103 g AlCl3
e.
molar mass SF6 = 146.1 g
4.9 104 mol SF6
146.1 g
1 mol
= 7.2 106 g SF6
f.
molar mass NH3 = 17.01 g
125 mol NH3
17.01 g
1 mol
= 2.13 103 g NH3
g.
molar mass Na2O2 = 77.98 g
0.01205 mol Na2O2
77.98 g
1 mol
= 0.9397 g Na2O2
81.
Before any calculations are done, the equations must be balanced.
a.
BaCl2(aq) + H2SO4(aq) BaSO4(s) + 2HCl(aq)
0.145 mol BaCl2
2
4
2
1 mol H SO
1 mol BaCl
= 0.145 mol H2SO4
b.
AgNO3(aq) + NaCl(aq) AgCl(s) + NaNO3(aq)
0.145 mol AgNO3
3
1 mol NaCl
1 mol AgNO
= 0.145 mol NaCl
c.
Pb(NO3)2(aq) + Na2CO3(aq) PbCO3(s) + 2NaNO3(aq)
0.145 mol Pb(NO3)2
2
3
3 2
1 mol Na CO
1 mol Pb(NO )
= 0.145 mol Na2CO3
d.
C3H8(g) + 5O2(g) 3CO2(g) + 4H2O(g)
0.145 mol C3H8
2
3
8
5 mol O
1 mol C H
= 0.725 mol O2
82.
2SO2(g) + O2(g) 2SO3(g)
molar masses: SO2, 64.07 g; SO3, 80.07 g; 150 kg = 1.5 105 g
1.5 105 g SO2
1 mol
64.07 g
= 2.34 103 mol SO2
194

Chapter 9: Chemical Quantities
2.34 103 mol SO2
3
2
2 mol SO
2 mol SO
= 2.34 103 mol SO3
2.34 103 mol SO3
80.07 g
1 mol
= 1.9 105 g SO3 = 1.9 102 kg SO3
83.
2ZnS(s) + 3O2(g) 2ZnO(s) + 2SO2(g)
molar masses: ZnS, 97.45 g; SO2, 64.07 g; 1.0 102 kg = 1.0 105 g
1.0 105 g ZnS
1 mol
97.45 g
= 1.026 103 mol ZnS
1.026 103 mol ZnS
2
2 mol SO
2 mol ZnS
= 1.026 103 mol SO2
1.026 103 mol SO2
64.07 g
1 mol
= 6.6 104 g SO2 = 66 kg SO2
84.
2Na2O2(s) + 2H2O(l) 4NaOH(aq) + O2(g)
molar masses: Na2O2, 77.98 g; O2, 32.00 g
3.25 g Na2O2
1 mol
77.98 g
= 0.0417 mol Na2O2
0.0417 mol Na2O2
2
2
2
1 mol O
2 mol Na O
= 0.0209 mol O2
0.0209 mol O2
32.00 g
1 mol
= 0.667 g O2
85.
Cu(s) + 2AgNO3(aq) Cu(NO3)2(aq) + 2Ag(s)
millimolar masses: Cu, 63.55 mg; AgNO3, 169.9 mg
1.95 mg AgNO3
1 mmol
169.9 mg
= 0.01148 mmol AgNO3
0.01148 mmol AgNO3
3
1 mmol Cu
2 mmol AgNO
= 0.005740 mmol Cu
0.005740 mmol Cu
63.55 g
1 mol
= 0.365 mg Cu
86.
Zn(s) + 2HCl(aq) ZnCl2(aq) + H2(g)
molar masses: Zn, 65.38 g; H2, 2.016 g
2.50 g Zn
1 mol
65.38 g
= 0.03824 mol Zn
0.03824 mol Zn
2
1 mol H
1 mol Zn
= 0.03824 mol H2
195

Chapter 9: Chemical Quantities
0.03824 mol H2
2.016 g
1 mol
= 0.0771 g H2
87.
2C2H2(g) + 5O2(g) 4CO2(g) + 2H2O(g)
molar masses: C2H2, 26.04 g; O2, 32.00 g; 150 g = 1.5 102 g
1.5 102 g C2H2
1 mol
26.04 g
= 5.760 mol C2H2
5.760 mol C2H2
2
2
2
5 mol O
2 mol C H
= 14.40 mol O2
14.40 mol O2
32.00 g
1 mol
= 4.6 102 g O2
88.
a.
2Na(s) + Br2(l) 2NaBr(s)
molar masses: Na, 22.99 g; Br2, 159.8 g; NaBr, 102.9 g
5.0 g Na
1 mol
22.99 g
= 0.2175 mol Na
5.0 g Br2
1 mol
159.8 g
= 0.03129 mol Br2
Intuitively, we would suspect that Br2 is the limiting reactant, because there is much less
Br2 than Na on a mole basis. To prove that Br2 is the limiting reactant, the following
calculation is needed:
0.03129 mol Br2
2
2 mol Na
1 mol Br
= 0.06258 mol Na.
Clearly, there is more Na than this present, so Br2 limits the reaction extent and the
amount of NaBr formed.
0.03129 mol Br2
2
2 mol NaBr
1 mol Br
= 0.06258 mol NaBr
0.06258 mol NaBr
102.9 g
1 mol
= 6.4 g NaBr
b.
Zn(s) + CuSO4(aq) ZnSO4(aq) + Cu(s)
molar masses: Zn, 65.38 g; Cu, 63.55 g; ZnSO4, 161.5 g; CuSO4, 159.6 g
5.0 g Zn
1 mol
65.38 g
= 0.07648 mol Zn
5.0 g CuSO4
1 mol
159.6 g
= 0.03132 mol CuSO4
As the coefficients of Zn and CuSO4 are the same in the balanced chemical equation, an
equal number of moles of Zn and CuSO4 would be needed for complete reaction. There is
less CuSO4 present, so CuSO4 must be the limiting reactant.
196
Chapter 9: Chemical Quantities
0.03132 mol CuSO4
4
4
1 mol ZnSO
1 mol CuSO
= 0.03132 mol ZnSO4
0.03132 mol ZnSO4
161.5 g
1 mol
= 5.1 g ZnSO4
0.03132 mol CuSO4
4
1 mol Cu
1 mol CuSO
= 0.03132 mol Cu
0.03132 mol Cu
63.55 g
1 mol
= 2.0 g Cu
c.
NH4Cl(aq) + NaOH(aq) NH3(g) + H2O(l) + NaCl(aq)
molar masses: NH4Cl, 53.49 g; NaOH, 40.00 g; NH3, 17.03 g; H2O, 18.02 g;
NaCl, 58.44 g
5.0 g NH4Cl
1 mol
53.49 g
= 0.09348 mol NH4Cl
5.0 g NaOH
1 mol
40.00 g
= 0.1250 mol NaOH
As the coefficients of NH4Cl and NaOH are both one in the balanced chemical equation
for the reaction, an equal number of moles of NH4Cl and NaOH would be needed for
complete reaction. There is less NH4Cl present, so NH4Cl must be the limiting reactant.
As the coefficients of the products in the balanced chemical equation are also all one, if
0.09348 mol of NH4Cl (the limiting reactant) reacts completely, then 0.09348 mol of
each product will be formed.
0.09348 mol NH3
17.03 g
1 mol
= 1.6 g NH3
0.09348 mol H2O
18.02 g
1 mol
= 1.7 g H2O
0.09348 mol NaCl
58.44 g
1 mol
= 5.5 g NaCl
d.
Fe2O3(s) + 3CO(g) 2Fe(s) + 3CO2(g)
molar masses: Fe2O3, 159.7 g; CO, 28.01 g; Fe, 55.85 g; CO2, 44.01 g
5.0 g Fe2O3
1 mol
159.7 g
= 0.03131 mol Fe2O3
5.0 g CO
1 mol
28.01 g
= 0.1785 mol CO
Because there is considerably less Fe2O3 than CO on a mole basis, let’s see if Fe2O3 is the
limiting reactant.
0.03131 mol Fe2O3
2
3
3 mol CO
1 mol Fe O
= 0.09393 mol CO
197
Chapter 9: Chemical Quantities
As there is 0.1785 mol of CO present, but we have determined that only 0.09393 mol CO
would be needed to react with all the Fe2O3 present, then Fe2O3 must be the limiting
reactant. CO is present in excess.
0.03131 mol Fe2O3
2
3
2 mol Fe
55.85 g Fe
1 mol Fe O
1 mol Fe
= 3.5 g Fe
0.03131 mol Fe2O3
2
2
2
3
2
3 mol CO
44.01 g CO
1 mol Fe O
1 mol CO
= 4.1 g CO2
89.
a.
C2H5OH(l) + 3O2(g) 2CO2(g) + 3H2O(l)
molar masses: C2H5OH, 46.07 g; O2, 32.00 g; CO2, 44.01 g
25.0 g C2H5OH
1 mol
46.07 g
= 0.5427 mol C2H5OH
25.0 g O2
1 mol
32.00 g
= 0.7813 mol O2
As there is less C2H5OH present on a mole basis, see if this substance is the limiting
reactant.
0.5427 mol C2H5OH
2
2
5
3 mol O
1 mol C H OH
= 1.6281 mol O2.
From the above calculation, C2H5OH must not be the limiting reactant (even though there
is a smaller number of moles of C2H5OH present) because more oxygen than is present
would be required to react completely with the C2H5OH present. Oxygen is the limiting
reactant.
0.7813 mol O2
2
2
2
2
2 mol CO
44.01 g CO
3 mol O
1 mol CO
= 22.9 g CO2
b.
N2(g) + O2(g) 2NO(g)
molar masses: N2, 28.02 g; O2, 32.00 g; NO, 30.01 g
25.0 g N2
1 mol
28.02 g
= 0.8922 mol N2
25.0 g O2
1 mol
32.00 g
= 0.7813 mol O2
As the coefficients of N2 and O2 are the same in the balanced chemical equation for the
reaction, an equal number of moles of each substance would be necessary for complete
reaction. There is less O2 present on a mole basis, so O2 must be the limiting reactant.
0.7813 mol O2
2 mol NO 30.01 g NO
1 mol O2
1 mol NO
= 46.9 g NO
c.
2NaClO2(aq) + Cl2(g) 2ClO2(g) + 2NaCl(aq)
molar masses: NaClO2, 90.44 g; Cl2, 70.90 g; NaCl, 58.44 g
198
Chapter 9: Chemical Quantities
25.0 g NaClO2
1 mol
90.44 g
= 0.2764 mol NaClO2
25.0 g Cl2
1 mol
70.90 g
= 0.3526 mol Cl2
See if NaClO2 is the limiting reactant.
0.2764 mol NaClO2
2
2
1 mol Cl
2 mol NaClO
= 0.1382 mol Cl2
As 0.2764 mol of NaClO2 would require only 0.1382 mol Cl2 to react completely (and
since we have more than this amount of Cl2), then NaClO2 must indeed be the limiting
reactant.
0.2764 mol NaClO2
2
2 mol NaCl
58.44 g NaCl
2 mol NaClO
1 mol NaCl
= 16.2 g NaCl
d.
3H2(g) + N2(g) 2NH3(g)
molar masses: H2, 2.016 g; N2, 28.02 g; NH3, 17.03 g
25.0 g H2
1 mol
2.016 g
= 12.40 mol H2
25.0 g N2
1 mol
28.02 g
= 0.8922 mol N2
See if N2 is the limiting reactant.
0.8922 mol N2
2
2
3mol H
1 mol N
= 2.677 mol H2
N2 is clearly the limiting reactant, because there is 12.40 mol H2 present (a large excess).
0.8922 mol N2
3
3
2
3
2 mol NH
17.03 g NH
1 mol N
1 mol NH
= 30.4 g NH3
90.
N2H4(l) + O2(g) N2(g) + 2H2O(g)
molar masses: N2H4, 32.05 g; O2, 32.00 g; N2, 28.02 g; H2O, 18.02 g
20.0 g N2H4
1 mol
32.05 g
= 0.624 mol N2H4
20.0 g O2
1 mol
32.00 g
= 0.625 mol O2
The two reactants are present in nearly the required ratio for complete reaction (due to the 1:1
stoichiometry of the reaction and the very similar molar masses of the substances). We will
consider N2H4 as the limiting reactant in the following calculations.
0.624 mol N2H4
2
2
2
4
2
1 mol N
28.02 g N
1 mol N H
1 mol N
= 17.5 g N2
199

Chapter 9: Chemical Quantities
0.624 mol N2H4
2
2
2
4
2
2 mol H O
18.02 g H O
1 mol N H
1 mol H O
= 22.5 g H2O
91.
a.
b.
92.
12.5 g theory
40 g actual
100 g theory
= 5.0 g
93.
C5H12(l) + 8O2(g) 5CO2(g) + 6H2O(l)
molar masses: C5H12, 72.146 g; H2O, 18.016 g
20.4 g C5H12
= 0.283 mol C5H12
0.283 mol C5H12
= 1.70 mol H2O
1.70 mol H2O
= 30.6 g H2O
94.
The balanced equation is: 2NaNO3 2NaNO2 + O2
0.2339 g NaNO2
= 0.003390 mol NaNO2
0.003390 mol NaNO2
= 0.003390 mol NaNO3
0.003390 mol NaNO3
= 0.28815 g NaNO3
% NaNO3 =
100 = 68.12%
200

Chapter 9: Chemical Quantities
95.
The balanced equation is: 2LiOH(s) + CO2(g) Li2CO3(s) + H2O(l)
67.4 g LiOH
= 2.81 mol LiOH
2.81 mol LiOH
= 1.41 mol Li2CO3
1.41 mol Li2CO3
= 104 g Li2CO3
96.
The balanced equation is: Fe2O3(s) + 2Al(s) 2Fe(l) + Al2O3(s)
a.
25.69 g Fe
= 0.4600 mol Fe
0.4600 mol Fe
= 0.2300 mol Fe2O3
0.2300 mol Fe2O3
= 36.73 g Fe2O3
b.
0.4600 mol Fe
= 0.4600 mol Al
0.4600 mol Al
= 12.41 g Al
c.
0.4600 mol Fe
= 0.2300 mol Al2O3
0.2300 mol Al2O3
= 23.45 g Al2O3
97.
2H2S(g) + 3O2(g) 2SO2(g) + 2H2O(g)
O2 is the limiting reactant (only 2.0 mol H2S needed).
3.0 mol O2
= 2.0 mol SO2
98.
The balanced equation is: 2NH3(g) + 2Na(s) 2NaNH2(s) + H2(g)
32.8 g NH3
= 1.93 mol NH3
16.6 g Na
= 0.722 mol Na
Na is the limiting reactant (only 0.722 mol NH3 needed).
201

Chapter 9: Chemical Quantities
0.722 mol Na
= 28.2 g NaNH2
0.722 mol Na
= 0.728 g H2
99.
The balanced equation is: SO2(g) + 2NaOH(s) Na2SO3(s) + H2O(l)
38.3 g SO2
= 0.598 mol SO2
32.8 g NaOH
= 0.820 mol NaOH
NaOH is the limiting reactant (only 0.410 mol SO2 needed).
0.820 mol NaOH
= 51.7 g Na2SO3
0.820 mol NaOH
= 7.39 g H2O
100.
2C3H6(g) + 2NH3(g) + 3O2(g) 2C3H3N(g) + 6H2O(g)
a.
5.23 102 g C3H6
= 12.4 mol C3H6
12.4 mol C3H6
= 658 g C3H3N
5.00 102 g NH3
= 29.4 mol NH3
29.4 mol NH3
= 1560 g C3H3N
1.00 103 g O2
= 31.25 mol O2
31.25 mol O2
= 1110 g C3H3N
Thus, C3H6 is the limiting reactant and 658 g C3H3N is produced (660. g C3H3N if
decimals are carried through).
b.
12.4 mol C3H6
= 670. g H2O (672 g H2O if decimals
are carried through)
c.
C3H6: 0 g (limiting reactant and is used up)
202

Chapter 9: Chemical Quantities
NH3:
12.4 mol C3H6
= 12.4 mol NH3 used up
29.4 mol NH3 – 12.4 mol NH3 = 17.0 mol NH3 leftover
17.0 mol NH3
= 290. g NH3 (289 g NH3 if decimals are carried
through)
O2:
12.4 mol C3H6
= 18.6 mol O2 used up
31.25 mol O2 – 18.6 mol O2 = 12.65 mol O2 leftover
12.65 mol O2
= 405 g O2
203